The Riemann Hypothesis is also equivalent to $|\pi(x) - Li(x)| = O(x^{1/2 + \epsilon})$, so let's look at that instead. In other words, $\log$ of the error should be about $(1/2) \log x$.
The sequence of points plotted below is $( \log x,\ \log |\pi(x) - Li(x)|)$ for $x=10^k$, with $1 \leq k \leq 23$. The straight line has slope $1/2$, with constant term chosen by a least squares fit (specifically, the line is $x/2 -1.24878$). Interpreted in this way, you can definitely see the promised asymptotic behavior.
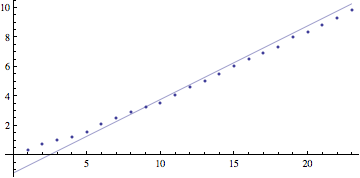 alt text http://www.math.lsa.umich.edu/%7Espeyer/PrimeError.png(source)
alt text http://www.math.lsa.umich.edu/%7Espeyer/PrimeError.png(source)
Data(Data set courtesy of Wikipedia.)
Note: My $\log$'s are base $10$, since my data set was binned by powers of $10$ already. Of course, that doesn't effect the slope.
