I assume you are aware that the vertex-transitive graphs represent a relatively constrained list? See this description. I fear that adding your conditions (2,3,4) on top of vertex-transitivity will again confine you to the skeletons of the Platonic solids.
Are you familiar with Fuller's geodesic domes?
They fail to satisfy your criteria, but they get perhaps
as close as is feasible, and may serve, depending upon your application:
Geodesic Dome http://cs.smith.edu/%7Eorourke/MathOverflow/GeodesicDome.jpg
After seeing your addendum on motivation, perhaps you should investigate the literature on packing disks on a sphere. For example, you might explore Neil Sloane's web pages on "[Nice arrangements of points on a sphere in various dimensions][4]" which address the problem of > placing $n$ points on a sphere in $d$ dimensions so as to maximize the minimal distance (or equivalently the minimal angle) between them.
For example, here is the best packing known for $n=50$, due to Székely (1974):
50 points on sphere http://cs.smith.edu/%7Eorourke/MathOverflow/pack50.jpg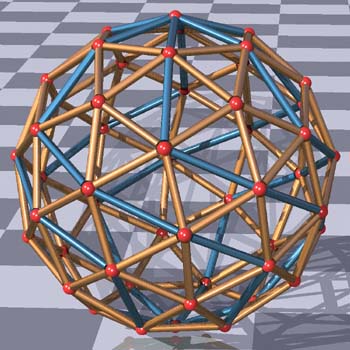
Image © Hugo Pfoertner, 2001
