Another proof of the sum of the first $n$ squares, relying on the knowledge of the formula for the sum of the first $n$ numbers:
$1^2 + 2^2 + \dots + n^2 = n(n+1)(2n+1)/6$$$1^2 + 2^2 + \dots + n^2 = n(n+1)(2n+1)/6$$
alt text http://jeremykun.files.wordpress.com/2011/06/triangle-proof.png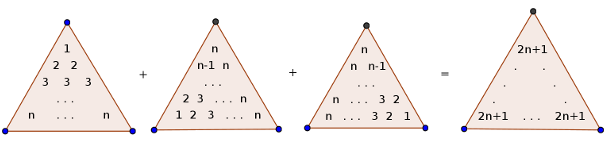
This one has a similar flavor to the fabled proof by Gauss of the sum of the first $n$ numbers. It's a good follow up for students after Gauss's proof.
