Let $S$ be a sphere in $\mathbb{R}^3$. Let $C$ be a closed curve in $\mathbb{R}^3$ disjoint from and exterior to $S$ which has the property that every point $x$ on $S$ is visible to some point $y$ of $C$, in the sense that the segment $xy$ intersects $S$ at precisely the one point $x$. I am interested in the shortest $C$ with this property. In computational geometry, such paths are called watchman tours, and there are many results concerning polygons in the plane finding such tours.
This question arose at a conference I'm attending, and I was pointed to a paper by V. A. Zalgaller:
"Shortest Inspection Curves for the Sphere" (Journal of Mathematical Sciences, Volume 131, Number 1, 5307-5320Journal of Mathematical Sciences, Volume 131, Number 1, 5307-5320; Translated from Zapiski Nauchnykh Seminarov POMI, Vol. 299, 2003, pp. 87–108.)
I cannot access the paper from the conference, but from the abstract it appears he focused on open rather than closed curves.
Has anyone heard of this natural question? Can you point me to relevant literature? Thanks!
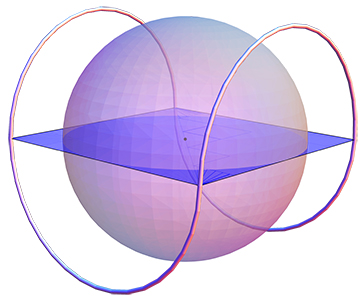
Addendum. Here is the $4\pi$ saddle / baseball-stitches curve suggested by Gjergji Zaimi: