Here's an example on the edgeless ${\bf Z}^2$ board that shows "mate in $3\omega$ moves" and, I think, can be extended to arbitrarily large multiples $N\omega$ by moving the outlying Knight about $N/2$ squares to the left. FEN = 14/5p4/5Pp3/4p1B1p1/N3p1Prrp/2p1p1prkp/2p1p1prpp/2PpN1B1np/3P1B3p/4P4p/9p/9p/9Pr/11K/1:
alt text http://math.harvard.edu/%7Eelkies/nomega.png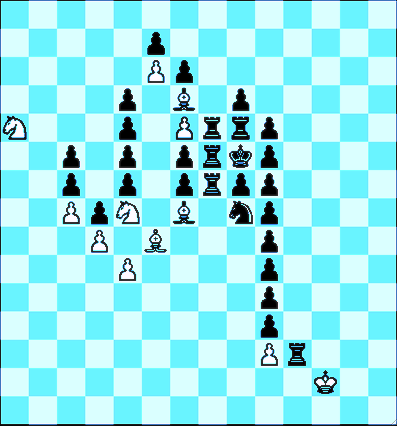
(source: harvard.edu)
Black to move. White will play Na11-b13-c11/d12, then capture the pawn on e10 (four squares left of the Black King), and checkmate with N(either)xg9+, Nxg9; Nxg9 exploiting the pin on the Rook at h9. Black gets three chances to move the Rook arbitrarily far to the right and harass the White King with horizontal checks until the King reaches the Rook.
Other Black defenses are no better, as long as White takes care not to move the King to one of the few squares where it could be checked with a move of the Black Knight. If that Knight moves without giving check then Nxg9 is mate immediately (which in turn means the White Knight on e7 must not move, else Black can unwind by moving the Knight, pushing the pawn to its square, and escaping with the King). None of the other Black pieces have a legal move except the Rook now on k3 (near the White King). If that Rook captures a pawn, White retakes with a pawn or Bishop and Black must soon move the Knight and get mated. Likewise after ...RxBf7; Pxf7, or ...RxBg8; Bxg8, or ...Rh12 (attacking Bg11); Pxh12. Finally if Black moves the Rook up and around to the d-file to answer Nc11 with Rd10 then White plays a random "waiting" move with his King and Black must move the Rook and allow Nxe10 and mate in two more moves.
Except for the Rook captures of the previous paragraph, White in turn must not move any piece except the King, the roving Knight, and possibly the Bf7 which must still be ready to return to f7 to defend the Bg8 and block the Rook's path to f8-f11. For example, after ...RxBf7 White must not play Bxf7? lest Black escape with ...g8; Bxg8, g9, followed by Rf10 (or g10), Kh10, etc.
To construct larger multiples of $\omega$, move the Knight from a11 far enough to the left. Black still has nothing better than to delay the King with horizontal checks. For instance, if Black could answer Nb13 with Rc12/d11 then White would play a random King move to force the Rook to relinquish its control over either c11 or d12 so the Knight could advance further. I think the Knight has enough freedom to reach its goal with the assistance of such waiting moves no matter what the Rook does. But if I'm wrong then a cavalry of $O(1)$ Knights would certainly suffice.
