I'm quite surprised no-one pointed out this one yet:
Theorem. The trefoil knot is knotted.
Proof.
alt text http://poisson.phc.unipi.it/%7Egolla/trefoil.png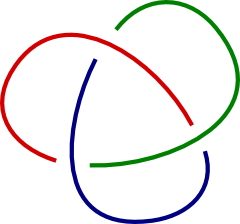 $\square$
$\square$
Some comments: a 3-colouring of a knot diagram D is a choice of one of three colours for each arc D, such that at each crossing one sees either all three colours or one single colour. Every diagram admits at least three colourings, i.e. the constant ones. We'll call nontrivial every 3-colouring in which at least two colours (and therefore all three) actually show up. It's easy to see (one theorem, more pictures!) that Reidemeister moves preserve the property of having a nontrivial 3-colouring, and that the unknot doesn't have any nontrivial colouring.
The picture shows a (nontrivial) 3-colouring of the trefoil.
EDIT: I've made explicit what "nontrivial" meant --― see comments below. Since I'm here, let me also point out that the number of 3-colourings is independent of the diagram, and is itself a knot invariant. It also happens to be a power of 3, and is related to the fundamental group of the knot complement (see Justin Robert's [Knot knotes] 23 if you're interested).
