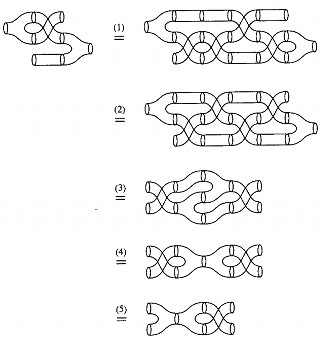
Algebraic manipulations in monoidal categories can also be performed in a graphical calculus. And the best part is that this is completely rigorous: a statement holds in the graphical language if and only if it holds (in the algebraic formulation). See for example Peter Selinger's "A survey of graphical languages for monoidal categoriesA survey of graphical languages for monoidal categories". There are many instances, for example in knot theory studied via braided categories. The following specific example comes from Joachim Kock's book "Frobenius Algebras and 2D Topological Quantum Field Theories"Frobenius Algebras and 2D Topological Quantum Field Theories", and proves that the comultiplication of a Frobenius algebra is cocommutative if and only if the multiplication is commutative.