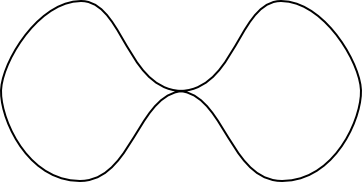
You can construct an immersion $\gamma$ which remains null-homotopic after removing any point $p$ not lying in its image. It suffices to let $\gamma$ travel along a graph in such a way that it runs along every edge the same number of times in each direction. As an example, you can take a train track with one central 4-valent switch and two arcs: it looks like an "8" but the 4-valent vertex is flattened, so that each of the two circles has a cusp:
[![The train track][1]][1]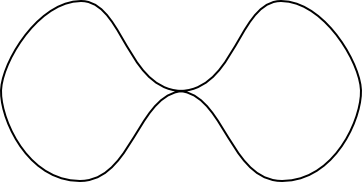
(source: unipi.it)
You can let a train travel along this train track so that it runs on each of the two arcs twice in opposite directions. [1]: https://i.sstatic.net/uZy0E.png