SLE - stochastic Loewner evolution (or Schramm-Loewner evolution) is a one parameter class of random planar curves. These random curves depend on a real parameter kappa, they are (almost suelysurely) simple curves when kappa is at most 4, they fill the plane when kappa is at least 8. They are related to many planar stochastic models. Look herehere for more pictures.
alt text http://www.math.nyu.edu/%7Esheff/SLE2.6666.bmp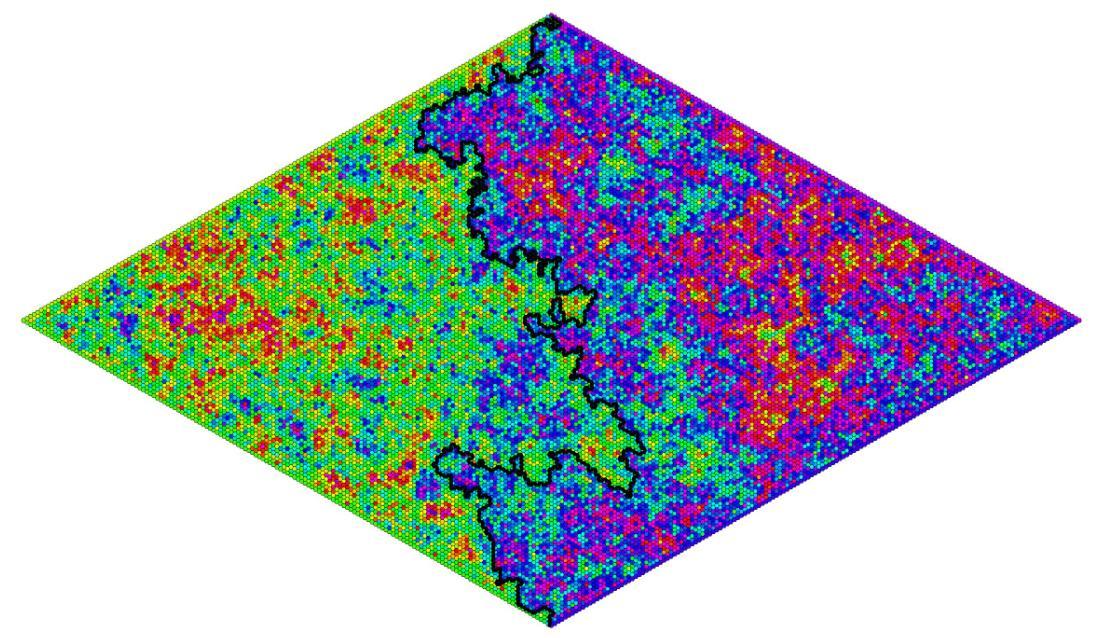 (source)
(source)
