This is slightly more mechanistic variant of Ian's response. When a knot is a Whitehead double, that means you've obtained it by doing a splicing construction (Larry Siebenmann's terminology, originally -- this is a particular formalism for describing satellite operations on knots, one that's friendly with the JSJ-decomposition for 3-manifolds). Alexander polynomials behave very nicely with respect to splicing, I'll describe it a bit below.
The input in a splicing construction is an $(n+1)$-component link $L=(L_0,L_1,\cdots,L_n)$ such that the sublink $(L_1,L_2,\cdots,L_n)$ is the trivial $n$-component link. The other input is $n$ knots $K_1,\cdots,K_n$. The splice knot I like to denote $L \bowtie K$. In the above Whithead double case, the input link $L$ is the Whitehead link, $n=1$ and the input knot $K$ is the figure-8 knot.
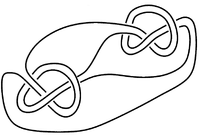
For the knot above, the input link $L$ has the same complement as the Borromean rings (but it isn't quite the Borromean rings). $n=2$ and the two knots are both trefoils (with the same handedness).
Alexander polynomials behave well under splicing, in particular:
$$\Delta_{L\bowtie K}(t) = \Delta_{L_0}(t) \Delta_{K_1}(t^{l_1}) \Delta_{K_2}(t^{l_2}) \cdots \Delta_{K_n}(t^{l_n})$$
where $l_i$ is the linking number between $L_i$ and $L_0$ in the link $L$.
So the "reason" the Alexander polynomial of a Whitehead double is trivial (from this perspective) is that (1) the linking numbers of the components of the Whitehead link are zero, and (2) the "base" component of the Whitehead link ($L_0$) has trivial Alexander polynomial (both components are unknots so they have to have trivial Alexander polynomials).
Anyhow, the proof of this is just the generalization of Agol's argument to this setting, by lifting everything to the abelianAbelian cover, Mayer-Vietoris type arguments.
But just staring at the above formula you see there are all kinds of other constructions on knots that produce new knots with trivial Alexander polynomials. More precisely, the example below is of a spliced knot which is the splice of the Borromean rings with two figure-8 knots, so its Alexander polynmomialpolynomial is trivial. There's no requirement to use figure-8 knots, any knots work but the way splicing works is you have to be quite careful about your framing conventions.
alt text http://s3.postimage.org/8eyzq3dox/blah2.jpg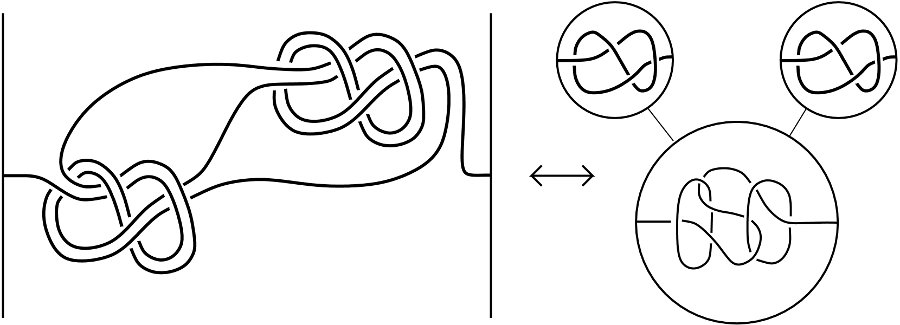 (source: Wayback Machine)
(source: Wayback Machine)
A diagram that indicates the framing conventions:
alt text http://s2.postimage.org/7h4kvwycx/blah3.jpg
So this knot also has trivial Alexander polynomial because $L_0$ is the unknot and all the respective linking numbers are zero.