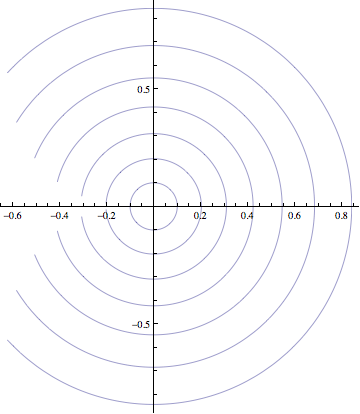
Let's roll the sphere, keeping the contact point on a line of constant lattitudelatitude $\phi$. (Here $\phi \in (0, \pi)$, with $\pi/2$ meaning the equator.) The path $b$ is an arc of a circle. The arc has radius $\tan \phi$, and sweeps out the angle $2 \pi \cos \phi$. So the distance from one end of the arc to the other is $2 (\tan \phi) \sin\left( \pi \cos \phi \right) = 2 O(\phi) \sin (\pi - O(\phi^2)) = O(\phi^3)$. So we see that the displacement goes to $0$ as $O(\phi^3)$, as desired.
broken images fixed (click 'rendered output' to see the difference); for more info, see https://meta.mathoverflow.net/a/4058/70594
Glorfindel
- 2.8k
- 6
- 28
- 38