Hilbert provedHilbert proved that there's no complete regular ($C^k$ for sufficiently large $k$) isometric embedding of the hyperbolic plane into $\mathbb{R}^3$. On the other hand, the pseudosphere is locally isometric to the hyperbolic plane up to its cusps (though it has the topology of a cylinder). What's the largest hyperbolic disk (with Gaussian curvature -1) that can be smoothly (or $C^2$, say) isometrically embedded in $\mathbb{R}^3$?
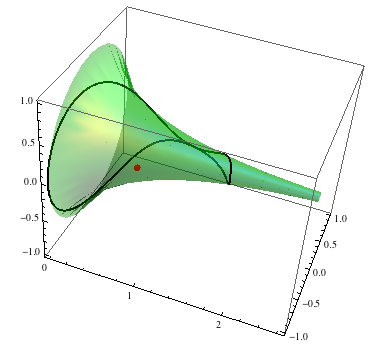
Edit: This doesn't seem to be getting many views, so I'll bump this by adding in a rather easy lower bound from the pseudosphere. First, the pseudosphere is parametrized by the region $$\mathrm{PS}=\{z \mid \mathrm{Im} z \ge 1,\; -\pi < Re z \le π\}$$ on the upper half-plane model of $H^2$. Let $z=x+iy$, so that ordered pairs $(x,y)∈ H^2$ when $y>0$.
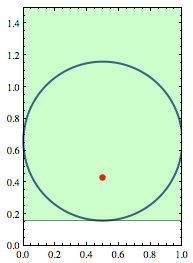
Next, Euclidean circles drawn on in the upper half-plane model with center $(x,y\cosh r)$ and radius $y\sinh r$ correspond to hyperbolic circles with center $(x,y)$ and radius $r$. I can fit a Euclidean circle of radius $π$ centered at $(0,1+π)$ into the region $\mathrm{PS}$. This corresponds to a hyperbolic disk of radius $\operatorname{arctanh}(π/(1+π)) \sim 0.993$.
Surely one can do better?
Edit 2: fixed mistakes in formulas above (didn't affect the bound). Here're some pictures: