There are many "dimensionless" ratios: choosing $R$ as the linear measurement is arbitrary. For instance, the ratio of the volume of the sphere to the volume of the circumscribed cube has a maximum at $n=1$. The ratio to the volume of the inscribed cube never attains a maximum. There are intermediate geometrically-related "midscribed" cubes, where all faces of some dimension are tangent to the unit sphere. Here is the graph for the ratio of volumes when the codimension 2 faces of a cube are tangent. It attains the maximum for $n = 12$ (just barely more than for $n=11$). There are many other reasonable dimensionless comparisons, for instance comparing to a simplex, etc. etc. Since the Gamma function grows super-exponentially, these simple geometric variations tend to shift the maximum --- there's nothing special about 5 or 7.
alt text http://dl.dropbox.com/u/5390048/MidScribed.jpg 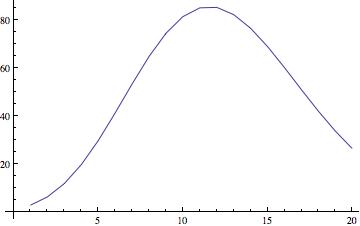 (source: Wayback Machine)
(source: Wayback Machine)
