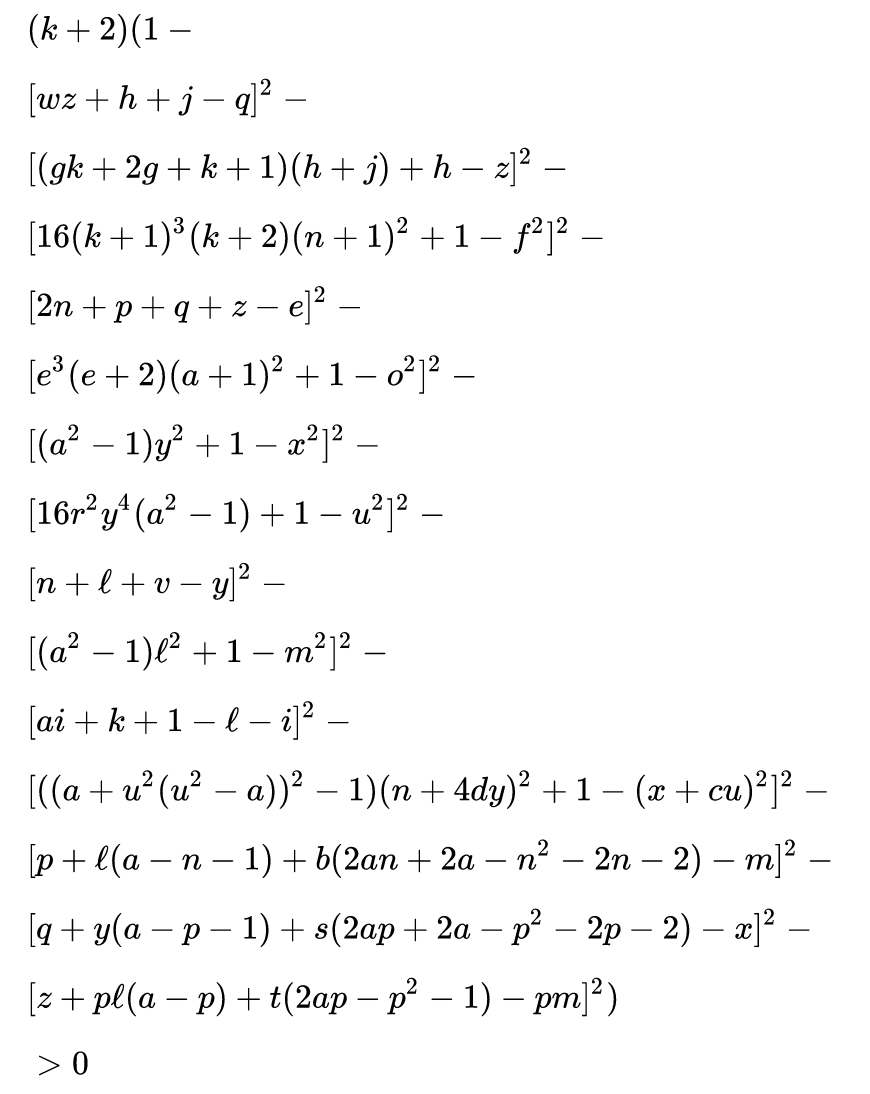If you accept polynomials in more than one variable...
There is a polynomial inequalityinequality in 26 variables that describes the set of primes :
(source: Wikipedia - from where I also sourced the rest of this answer). By a result of Matiyasevich there is another polynomial inequality in 10 variables that also describes the primes, but whose order in on the order ofis approximately $10^{45}$, which dwarfs Wadim's answer. In the other direction there is an order 4 polynomial inequality that will do the job, but in 58 variables.