This is the same question as here in SE.
I have a conjecture, it is like this:
Suppose there is a non-self-intersecting polygon lies inside a closed square of length $1$. The polygon has every side length of $1$. Suppose the polygon is not the boundary of the unit square (i.e. a square frame.) Prove or disprove that the number of sides of the polygon is an odd number.
I have pondered this problem for quite a while. What I have tried is this: Suppose the unit square is dissected into four $1/2\times 1/2$ quadrants, like $\begin{array}{|c|c|}\hline1 & 2\\\hline3&4\\\hline\end{array}$. Therefore, we want to prove that the line segments that go through the quadrants diagonally ($1$ to $4$, or $2$ to $3$) is odd number. To prove this, I want to prove the intermediate steps:
(1) We don't have both kinds of diagonals. That is, no edges from $1$ to $4$ and $2$ to $3$.
Update (from comments:) we may need to use the fact that it is a polygon. Generally If we place the segments in a good way, there can be two segments such that one segment has end points in regions $1$, $4$, And the other can have the segment has endpoint in regions $2$, $3$. For example, the segment connects $(0,0)$ and $(\sqrt 3/2−\epsilon_1,1/2+\epsilon_2)$; and the segment connects $(1−\sqrt 3/2,1)$ to $(1−\epsilon_3,1/2−\epsilon_4)$ for some small $\epsilon$'s (There does not need to be the same $\epsilon$.)
(2) All the diagonal-crossing segments are together.
(3) The diagonal crossing segment has odd number of them.
However, I stuck at all of them... Is there a way to solve this problem (even if not using the ideas from above)? Any help are welcome!
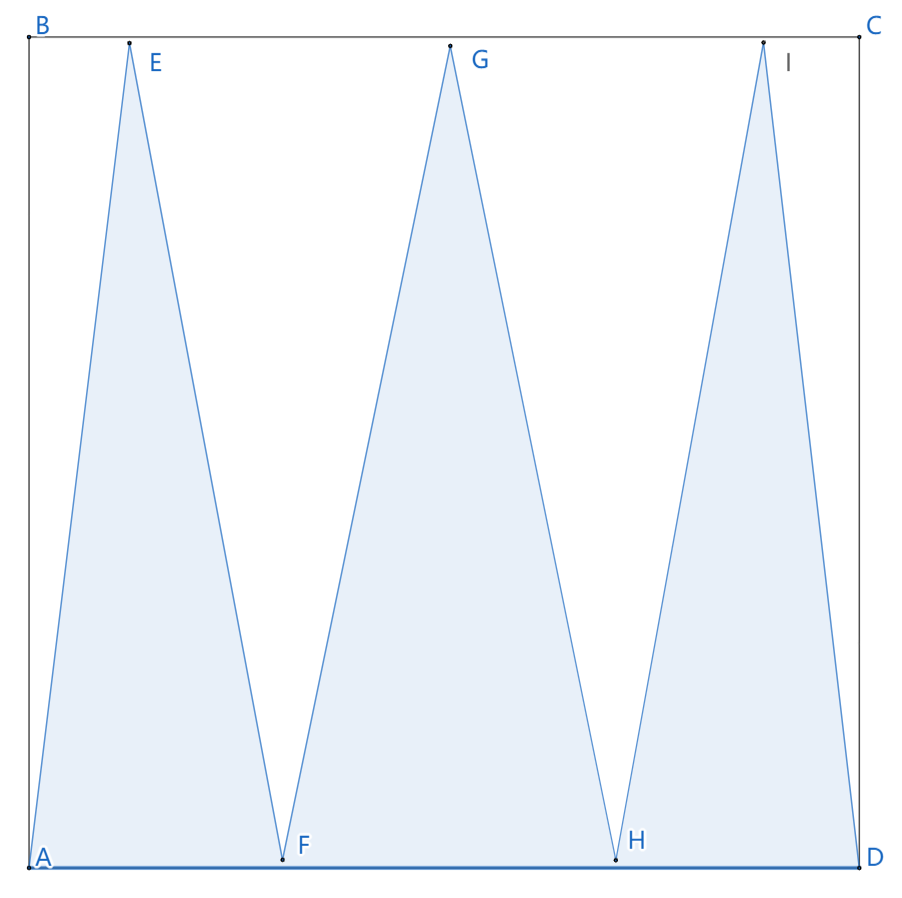
P.S. we can construct the polygon as this for $2k+1$ sides, as the zigzag shape:
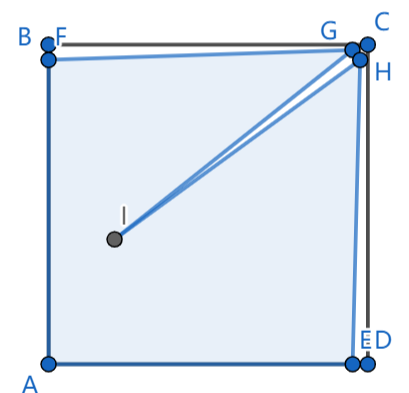
The square of size $1$ is the smallest possible, because we can have this shape for any square $1+\varepsilon$: we have the edges as the original square's edge and have a spike inside.
P.S. Someone the MSE suggested that we can divide the square into nine regions, instead of four in the problem...