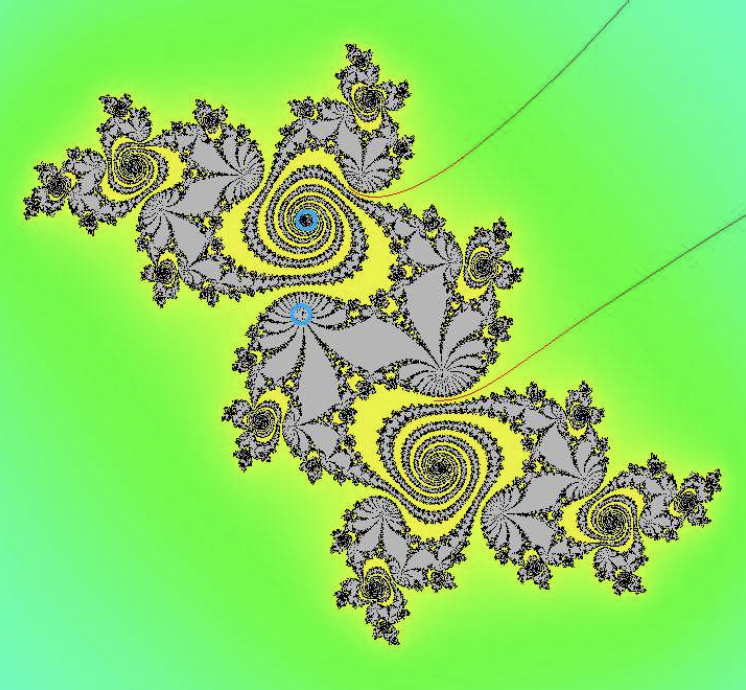
(More of a comment, but making this an answer to include an image.)
One nice reference is these slides from Milnor, which include theorems about non-locally-connected Julia sets, and some specific visualizations based on an iterated process. Here's an image from the slides that gives an intuition for what such Julia sets look like. (As
As Milnor points out, any visualization is just going to be an approximation. But if you examine the spirals, of course!)it makes sense this isn't a locally connected set in the limit.