$\newcommand\al\alpha\newcommand\de\delta\newcommand\R{\Bbb R}\newcommand\B{\mathrm B}$It follows from the ODE $$f''=f^{-\al} \tag{1}\label{10} $$ and the condition $f>0$ that $f$ is (strictly) convex. If $f(\infty-)<\infty$, then, by \eqref{10}, $f''(\infty-)>0$ and hence $f(\infty-)=\infty$. So, $f(\infty-)=\infty$. Similarly, $f(-\infty+)=\infty$. So, $\inf f=f(t_0)$ for some real $t_0$. By shifting, without loss of generality $t_0=0$.
Now take any real $\de>0$ and let $$g(z):=\int_\de^z\frac{dy}{\sqrt{k(\de^{1-\al}-y^{1-\al})}} \Big[=\frac{\de ^{(\al +1)/2} } {\sqrt{2} \sqrt{\al -1}}\, \B_{1-(z/\de)^{1-\al}}\Big(\frac{1}{2},\frac{1}{1-\al }\Big)\Big]$$$$g(z):=\int_\de^z\frac{dy}{\sqrt{k(\de^{1-\al}-y^{1-\al})}} \Big[=\frac{\de ^{(\al +1)/2} } {\sqrt{2} \sqrt{\al -1}}\, \B\Big(1-\Big(\frac z\de\Big)^{1-\al};\frac{1}{2},\frac{1}{1-\al }\Big)\Big]$$ for real $z\ge\de$, where $k:=\frac2{\al-1}$ and $\B$ is the incomplete beta function. Then $g\colon[\de,\infty)\to\R$ is a continuous function strictly increasing from $0$ to $\infty$ on the interval $[\de,\infty)$, and $g$ is smooth on the interval $(\de,\infty)$, whereas $g'(\de+)=\infty$.
For real $t$, let $f(t):=g^{-1}(|t|)$. Then $f$ is a solution to ODE \eqref{10} with $\inf f=f(0)=\de$ (see the Detail below). So, $f$ is the only solution of \eqref{10} satisfying the initial conditions $f(0)=\de$ and $f'(0)=0$.
Moreover, $|f'|=\sqrt{k(\de^{1-\al}-f^{1-\al})}\le\sqrt{k\de^{1-\al}}$, so that $f$ is Lipschitz and hence locally Hölder. However, this solution $f$ of ODE \eqref{10}, which is unique up to the shifting (given $\inf f$ ), is not globally Hölder with any exponent $<1$, because $f'(\infty-)=\sqrt{k\de^{1-\al}}>0$. So, there is no solution $f>0$ of \eqref{10} that globally Hölder with any exponent $<1$.
Detail: On the interval $[0,\infty)$, we have $f'=\sqrt{k(\de^{1-\al}-f^{1-\al})}$ and hence $$f''=\frac{-k(1-\al)f^{-\al}f'}{2\sqrt{k(\de^{1-\al}-f^{1-\al})}} =f^{-\al},$$ so that $f$ satisfies your ODE\eqref{10} holds on the interval $[0,\infty)$. Similarly, $f$ satisfies your ODE\eqref{10} holds on the interval $(-\infty,0]$.
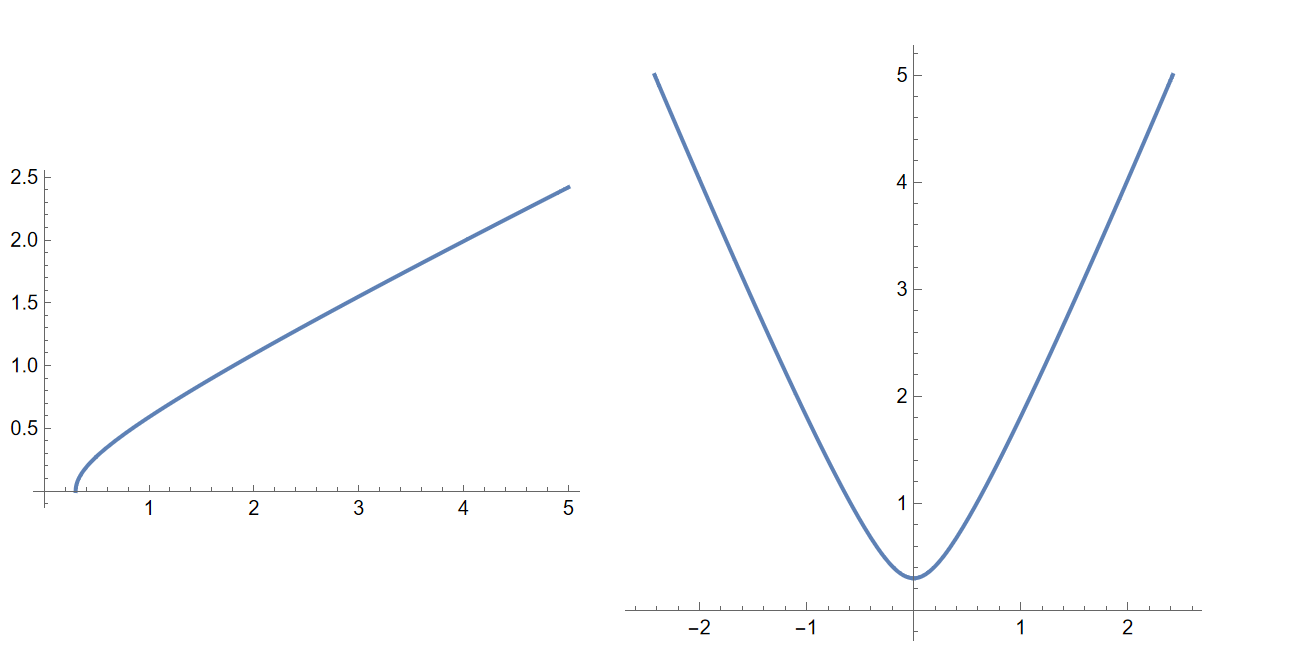
For an illustration, with $\al=3/2$ and $\de=3/10$, here are the graphs $\{(z,g(z))\colon\de\le z\le5\}$ (left) and $\{(t,f(t))\colon|t|\le g(5)\}$ (right):