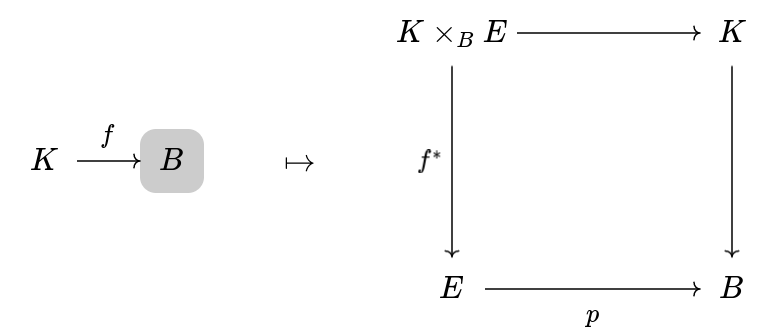Consider a functor $p \colon E \to B$ in $\mathbf{Cat}$. Then there is an induced functor $p^* \colon \mathbf{Cat}/B \to \mathbf{Cat}/E$. It is on objects given by
But now I wanna ask its possible to investigate using the domain functor $d^*\colon \mathbf{Cat}/E \to \mathbf{Cat}$ that to say that $p^*$ has a right adjoint is equivalent to saying that the functor $- \times_B E \colon \mathbf{Cat}/B \to \mathbf{Cat}$ has a right adjoint. Is this true? Is this just using the composition of adjunctions?