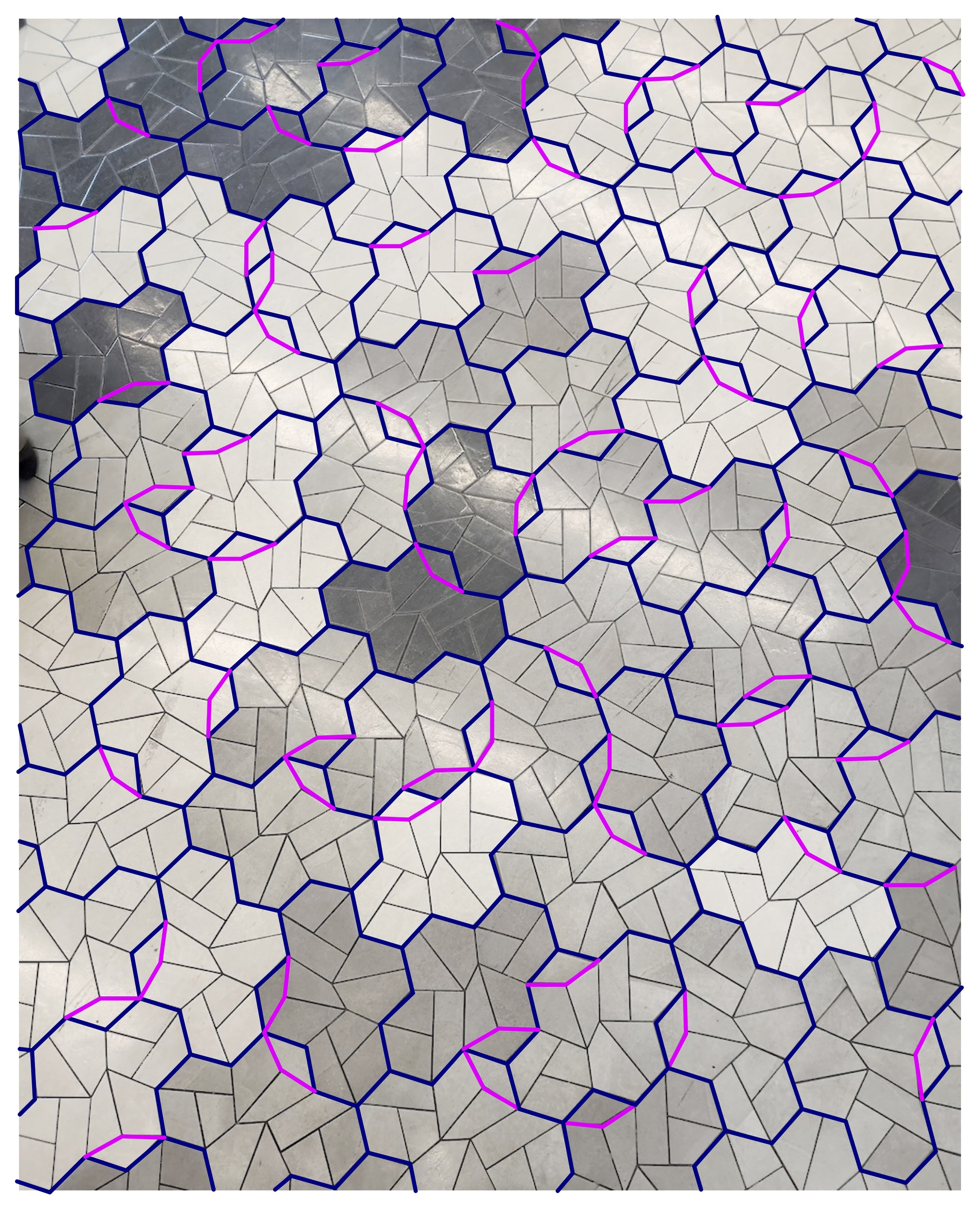
You can view this pattern as consisting of major and minor tiles. The major tiles are the union of four hexagons. These tiles are all identically subdivided into eleven minor tiles.
In the picture below the edges of the major tiles are highlighted in blue. The pink lines are one type of edge of the minor tiles. When they line up in a certain way, these give rise to the arcs observed in the question.
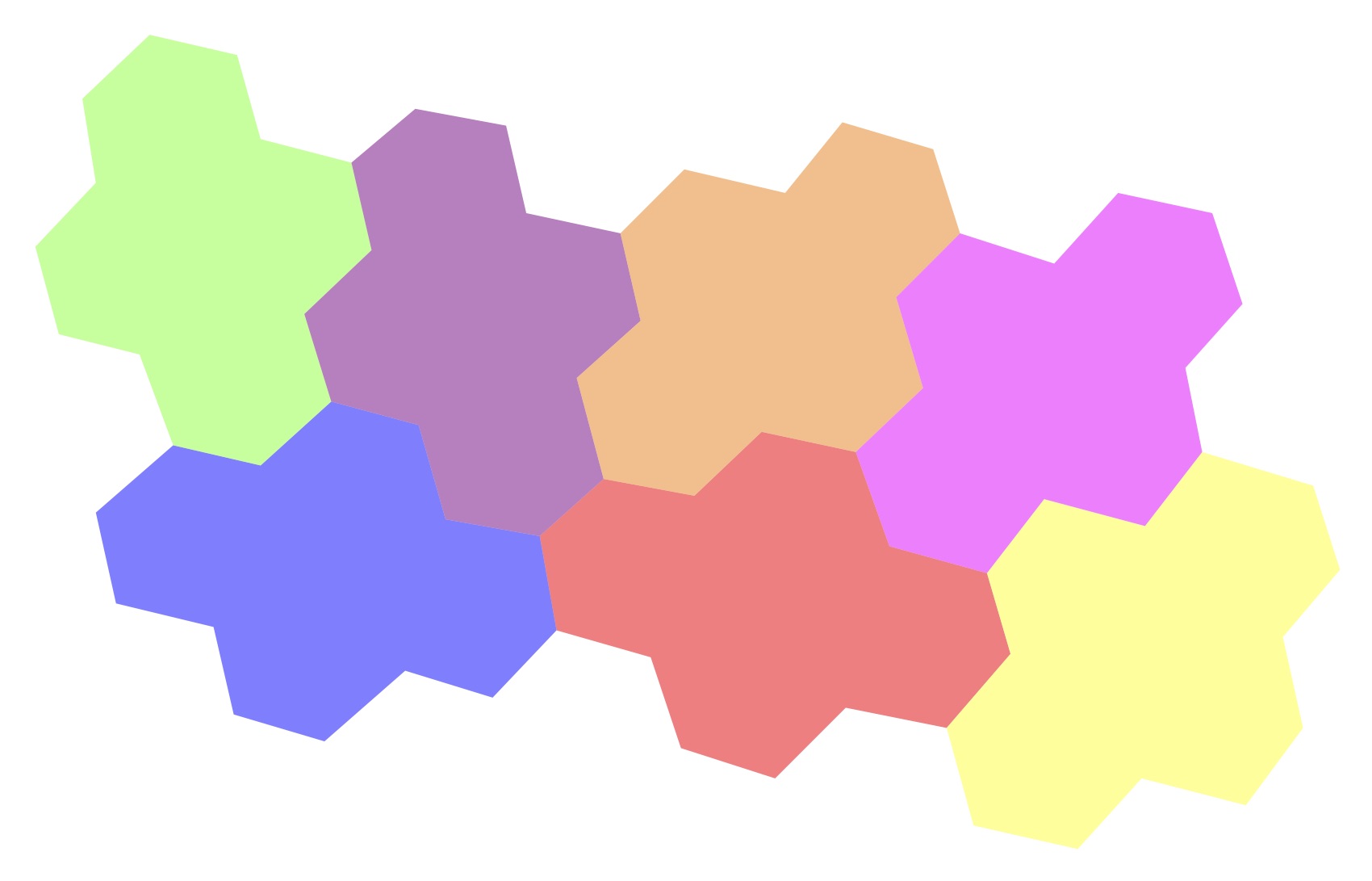
Ignoring the minor tiles, the tiling is periodic. The fundamental domain consists of seven major tiles:
The distribution of the pink edges in the previous picture suggests that the orientations of the major tiles (there are two possibilities for each one) are random. And finally, here is the original picture with the same coloring overlaid: