Consider the infinity category of simplicial rings $CAlg=Fun^{\prod}(Poly^{op},Spc)$, and the under-category over $R$: $CAlg_{A/}$ is equivalent to $CAlg_{A}=Fun^{\prod}(Poly^{op}_{R},Spc)$ by 25.1.4.3 and 25.1.4.4 in SAG by Lurie. And there is a final object $*$ in $CAlg$, which is the 'zero ring', the functor valued in the final object $*\in Set\hookrightarrow Spc$. As the property of under category, we can see that this zero ring $*$ is again a final object in $CAlg_{A}$.
I can see there is much development about the spectrum of the over-category $CAlg_{/A}$, for example, in 25.3.3 in SAG or 7.3.4 in HA. For the over-category $CAlg_{/A}$, the final object is the $id_{A}$ and the pointed objects of $(CAlg_{/A})_{*}=(CAlg_{/A})_{A/}$ is equivalent to $CAlg(Mod(A))_{/A}$ by 3.4.17 in HA, and this is exactly the 'augmented algebra in $Mod(A)$' as defined in definition 7.3.4.3 HA, which is in the shape like the following triangle: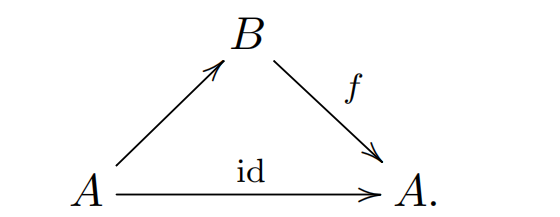
And we can show that the spectrum of this infinity category of augmented algebra is exactly the stable infinity category $Mod(A)$. Thus we get the spectrum of the over-category.
I can't help thinking about the dual case:
$\textbf{Question:}$ $\textbf{What is the spectrum object of the infinity category of simplicial commutative rings over $A$?}$
Note that we used the fact that $Sp(C)\cong Sp(C_{*})$ above, where $C=CAlg_{/A}$. So I also imitate this in the case when $C=CAlg_{A/}$, if we consider the pointed objects in $CAlg_{A}$, that is, a morphism from final object $*$ to $A$. Note that a discrete ring admitting a pointed morphism is exactly the zero ring, however, in the derived setting, the condition for the existence of a morphism(natural transformation) between simplicial rings(functors) boils down to the homotopy commutativity in $\textbf{Spc}$, unwinding these diagrams leads to the conclusion: the pointed objects in $CAlg_{A}$ are $A$ rings with $\pi_{0}$ trivial, that is, the path connected $A$ rings. This is indeed a interesting fact, but it doesn't help solve the problem.
Any ideas or thoughts on the spectrum object of $CAlg_{A}$ is very welcome.
$\textbf{Remark}$: As suggested by comments by Maxime Ramiz, indeed, if $\pi_{0}(A)$ is 0, then the whole simplicial ring is 0, because we have $\pi_{k}(A)$ as $\pi_{0}(A)$ module, which is zero because the only module over zero ring is zero ring itself. But indeed it's a very $\textbf{strange}$ thing that stablization of over-category $CAlg_{A}$ is only a singleton?
Moreover, if we consider not only the 'connected infinity ring' (the simplicial rings are actually connected if we consider their underlying $E_{\infty}$ rings), $CAlg_{{E_{\infty}}_{A/}}\cong CAlg_{{E_{\infty}}_{A//*}}\cong Mod(*)$$Sp(CAlg_{{E_{\infty}}_{A/}})\cong Sp(CAlg_{{E_{\infty}}_{A//*}})\cong Mod(*)$, where the infinity category of module spectra over zero ring $*$ is equivalent to the infinity derived category of discrete modules over a zero ring, again, there is nothing in it.
