Added) A few more words about polynomials for which the desired property fails. My argument for the claim below is admittedly hand-wavy at certain points; but I leave it here if someone can make it more precise.
Claim) Let $p(z)$ be a polynomial of degree $d\geq 2$ whose filled Julia set, denoted by $K$, is connected. Suppose there exist distinct points $p_1,\dots,p_N\in K$ such that the only connected subset of $K$ containing all of them is $K$ itself. Then:
- The Julia set, $\mathcal{J}$, and the filled Julia set, $K$, coincide and are homeomorphic to an interval.
- Every finite critical point is simple and belongs to the Julia set. Moreover, the critical values lie in a forward-invariant set of size two which does not contain any critical points.
In particular, when $d=2$, $p(z)$ must be conjugate to the Chebyshev polynomial $z^2-2$.
This is in a sense the converse of the Chebyshev polynomial example I began with: polynomials for which the desired property fails "resemble" Chebyshev polynomials.
$\underline{\text{I base my argument on the following two }``\text{facts"}}.$ I believe they are true but I couldn't find rigorously written proofs. Any reference on them is welcome.
"Fact" 1) If the filled Julia set, and hence the Julia set, are connected, then they are path connected.
I didn't find a proof in standard complex dynamics textbooks. If this is false, one can strengthen the assumption from the connectedness of the filled Julia set to its path-connectedness.
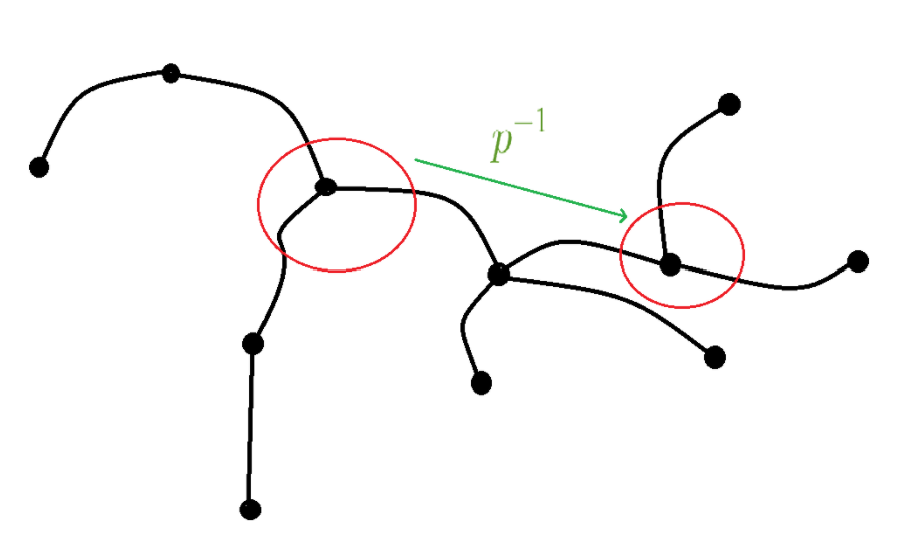
"Fact" 2) Let $X$ be a compact, path-connected metric space. Suppose there exist an integer $N\geq 2$ and distinct points $p_1,\dots,p_N\in X$ such that no proper path-connected subset of $X$ contains all $p_i$'s. Then, $X$ is homeomorphic to a tree with at most $N$ leaves.
I have posed this as a separate MO question.
Proof) Since the filled Julia set, $K$, is connected, it must contain all finite critical points. By Fact 1 above, $K$ is path-connected as well. Due to the existence of $p_1,\dots,p_N$, as mentioned before, there is no bounded Fatou component ($K$ has no interior points): Otherwise, one can remove a small enough open disk from $K$ to obtain a connected subset containing all $p_i$'s. Thus $K$ coincides with the Julia set $\mathcal{J}=\partial K$. Applying Fact 2 to the path-connected, compact space $\mathcal{J}$, we deduce that $\mathcal{J}$ is homeomorphic to a finite tree.
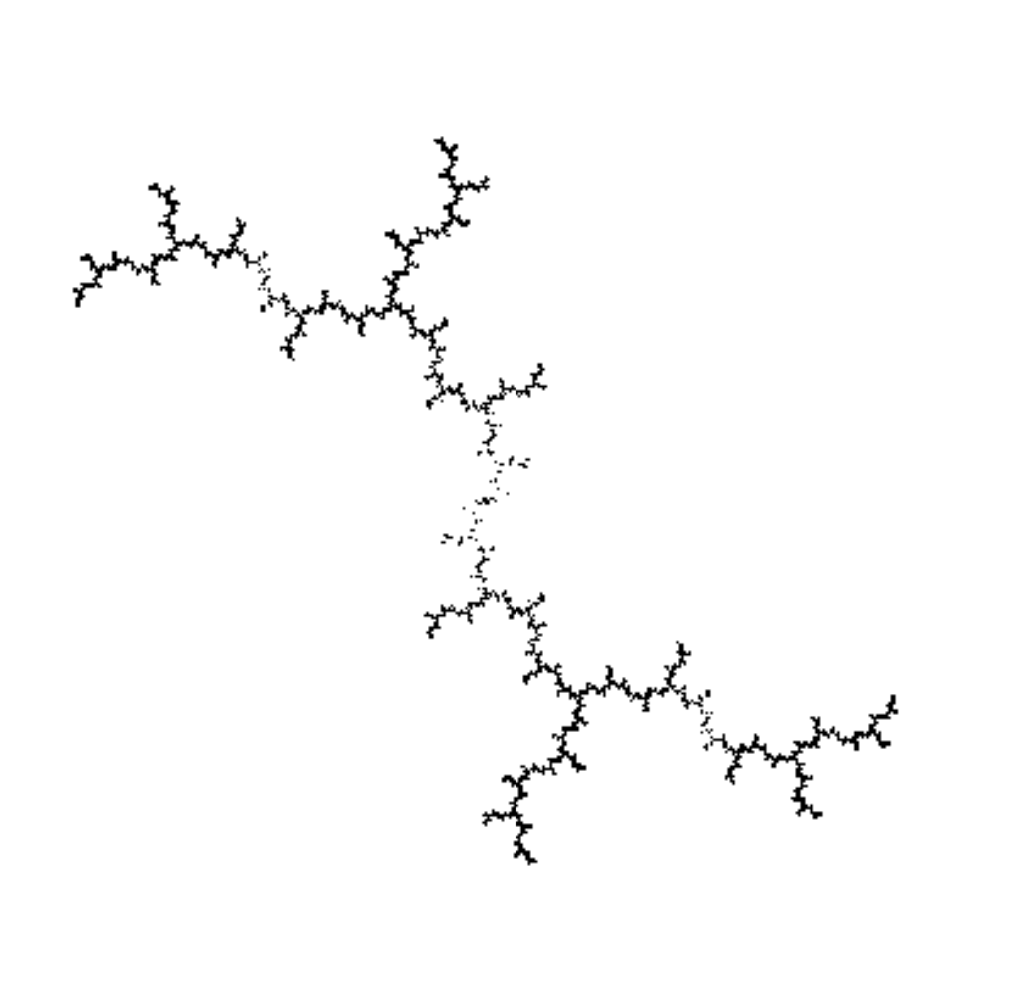
There are indeed polynomial Julia sets which are "tree-like", for instance the dendrite Julia set of $z\mapsto z^2+i$. (The picture was generated by this JavaScript app.)
But the example above has infinitely many "branch points", meaning points which do not admit a neighborhood homeomorphic to a closed/half-closed interval. I argue that if the Julia set is a finite tree (i.e. the number of leaves is finite), then there are no branch points, meaning $\mathcal{J}$ is homeomorphic to a closed interval. First, notice that since the Julia set is both backward and forward invariant, it makes sense to speak of the multiplicity of the restriction $p\restriction_{\mathcal{J}}:\mathcal{J}\rightarrow\mathcal{J}$ the way one considers the multiplicity of $p:\hat{\Bbb{C}}\rightarrow\hat{\Bbb{C}}$ at a point; and the restriction is a ramified cover of degree $d$. Any preimage of a branch point of tree $\mathcal{J}$ under $p$ should be a branch point as well. This is clear if $p$ is a local homeomorphism near the preimage point. If the preimage point is a critical point, then $p$ is many-to-one in its vicinity, and hence even more branches emanate from it.
On the other hand, the tree is finite, thus the branch points (vertices of degree $>2$) of $\mathcal{J}$, if any, constitute a non-empty finite subset $E$ of $\Bbb{C}$ with $p^{-1}(E)\subseteq E$. Such a polynomial is conjugate to $z\mapsto z^d$ (This follows from Riemann-Hurwitz theorem. As far as I recall, it is proved in Silverman's book.) But the filled Julia set is a disk in that case. We deduce that there is no branch point, and the Julia set $\mathcal{J}$ is homeomorphic to an interval.
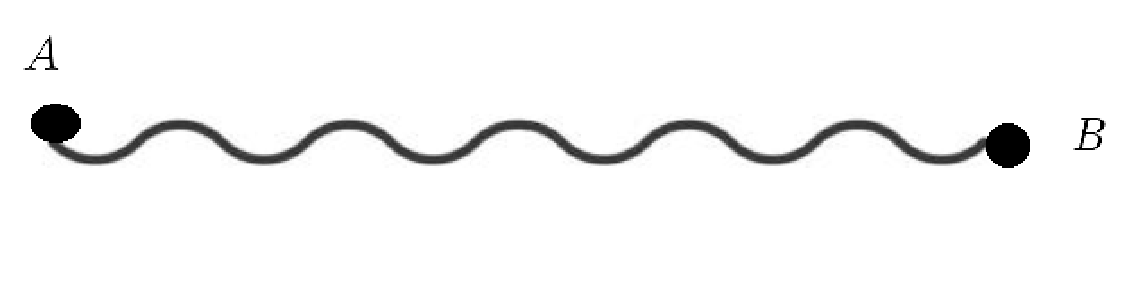
Keep in mind that $p\restriction_{\mathcal{J}}:\mathcal{J}\rightarrow\mathcal{J}$ is a ramified cover of degree $d$. The only points with neighborhoods homeomorphic to a half-closed interval are the endpoints, denoted by $A,B$ in the picture above. We deduce that $\{A,B\}$ is forward-invariant; and furthermore, every finite critical point of $p$ (we know that they all belong to $\mathcal{J}$) lies in the interior of $\mathcal{J}$, and is mapped to either $A$ or $B$ with multiplicity two. (In other words, under $p\restriction_{\mathcal{J}}$, the image of a neighborhood of a critical point, homeomorphic to an open interval, is homeomorphic to a half-closed interval, thus a neighborhood of either $A$ or $B$).
Finally, as a corollary, we show that when $d=2$ the polynomial $p(z)$ can be turned into $z\mapsto z^2-2$ by a linear change of coordinates. Write the polynomial in the normal form $p(z)=z^2+c$. The critical value $c$ must lie in an invariant set of size two. If $p(c)=c$, then $c$ should be zero---impossible because the filled Julia set of $p(z)=z^2$ is a disk, not a topological interval. That invariant set should therefore be $\{c,p(c)=c^2+c\}$. Now there are two possibilities:
- $p(c^2+c)=c^2+c\Leftrightarrow (c^2+c)^2=c^2\Leftrightarrow c\in\{0,-2\}$. The case of $c=0$ was ruled out before; and $c=-2$ yields the Chebyshev polynomial.
- $p(c^2+c)=c\Leftrightarrow c^2+c=0\Leftrightarrow c\in\{0,-1\}$. Again, $c=0$ is impossible. If $c=-1$, then the critical point $z=0$ lies in a $2$-cycle $0\mapsto -1\mapsto 0$. But there cannot be any finite super attracting cycle because its immediate basin of attraction determines a bounded Fatou component. (The Julia set of $z\mapsto z^2-1$ is the basilica Julia set, definitely not homeomorphic to an interval.)
Further questions) I wonder if there is an elegant argument for showing that a polynomial whose Julia set is homeomorphic to a closed interval must be Chebyshev. Stronger properties that we derived above (critical points are simple, critical values are periodic etc.) may also be used to establish such a thing because they are all properties of Chebyshev polynomials.