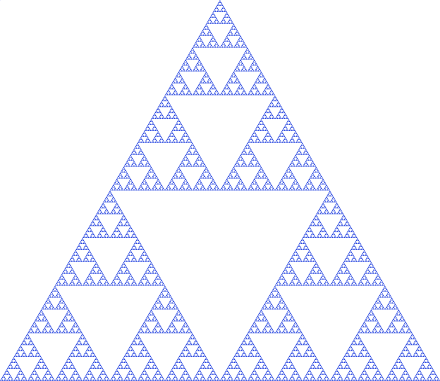
Consider the problem of packing an upwards-pointing unit equilateral triangle "efficiently" by downwards-pointing equilateral triangles, where "efficiently" means that there is little wasted area relative to the perimeter of the triangles used in the packing. The $n^{th}$ generation of the Sierpinski triangle
packs all but $(3/4)^n$ of the area of the large upwards triangle by downwards triangles, at the cost of a net perimeter of $\asymp (3/2)^n$. Thus, if we let $\varepsilon$ denote the area not packed, then the perimeter of the triangles used in this construction is $\gg \varepsilon^{-\alpha}$ for $\alpha = \frac{\log (3/2)}{\log (4/3)} = 1.409\dots$.
My question is whether this phenomenon is general: given any finite collection of downward equilateral triangles in the upward unit equilateral triangle that is a packing (i.e., interiors are disjoint) and leaves an area of $\varepsilon$ not covered, is it true that the total perimeter of the triangles used is of the form $\gg \varepsilon^{-c}$ for some absolute constant $c>0$? For my application I do not need an optimal exponent $c$. [EDIT: as pointed out in answers, to make the answer positive for large $\varepsilon$, the outer triangle should also count towards the total perimeter.]
I think I can establish a bound of the form $\gg \log \frac{1}{\varepsilon}$ (roughly speaking, by arguing that every dyadic scale of triangles between $\varepsilon$ and $1$ has to contribute a constant amount of perimeter, otherwise there will be too much waste), but for my application I really need a polynomial lower bound (or maybe $\exp( (\log\log \frac{1}{\varepsilon})^C )$ for a large absolute constant $C$ might suffice). It's intuitively plausible to me that the Sierpinski packing is the "best" packing for this purpose, and that the smaller triangles really have to contribute more than a constant amount of perimeter, but I am finding it surprisingly tricky to locate a rigorous argument. Perhaps this sort of question has already been studied in the literature?
One can interpret this question as an exotic form of an isoperimetric inequality, where the region of interest is required to be a disjoint union of downwards pointing equilateral triangles in a fixed upward pointing triangle, but this question seems rather orthogonal to the usual theory of isoperimetric inequalities, so I don't believe that this interpretation is particularly fruitful.