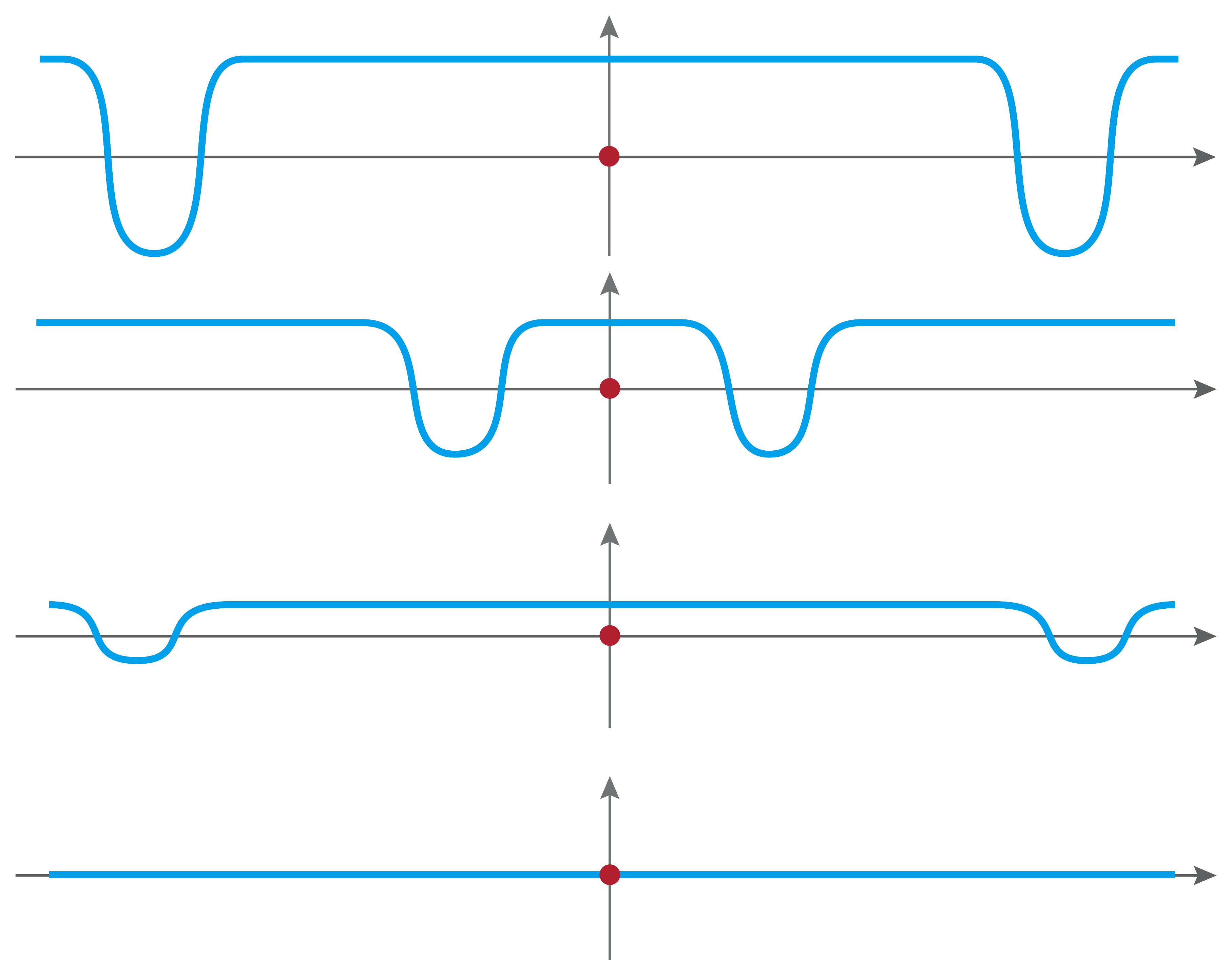
Here is an example which shows that the answer is no. Each $\Gamma_t$ is the graph of a smooth symmetric function on $[-1,1]$. For $t\in[0,1)$, $\Gamma_t$ lies above the $x$-axis except for a pair of bumps which descend below it. As $t\to 1$, the position of each of the bumps keeps oscillating to the right and left, faster and faster, while remaining on its respective side of the $y$-axis. At the same time the height of $\Gamma_t$ shrinks to $0$ and it converges to $[-1,1]$, which is defined to be $\Gamma_1$.
Any selection of points $x_i(t)\in\Gamma_t$ to contain $o$ within their convex hull must include a point from the portion of each of the bumps on or below the $x$-axis. As the bumps constantly move side to side (by a distance bigger than the width of each bump) the first coordinates of these points are forced to oscillate indefinitely and hence cannot converge to unique limit points as $t\to 1$. So $x_i(t)$ cannot be chosen continuously for all $t\in[0,1]$.