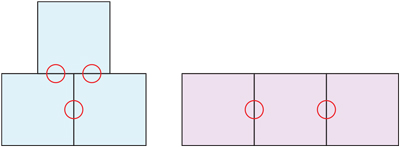
The left shape below has $3$ contacts (circled) "between pairs of units" and hull area $> 3$, while the right shape has $2$ contacts and area $3$. So minimizing the hull area does not always maximize contacts.
(After OP's edit.) I don't think minimizing perimeter will maximize contacts, either. Here is an idea for a proof (but not a proof). The left image below show the optimal packing of $10$ congruent disks in a circle, from this Wikipedia article. I count $12$ contacts. Cannonball packing $5$ disks in two rows has many more contacts---$17$. The circumscribing circle has a much smaller circumference doesthan the perimeter of the bounding rectangle of the two rows of five disks. It seems plausible that using the convex hull instead would not change the situation: the perimeter of the hull of the disk packing on the left is likely smaller than the hull on the right. But I emphasize I did not verify this through a careful calculation.