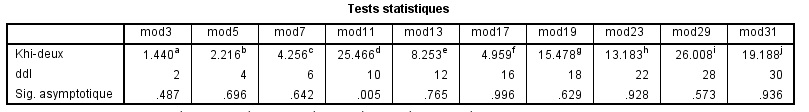
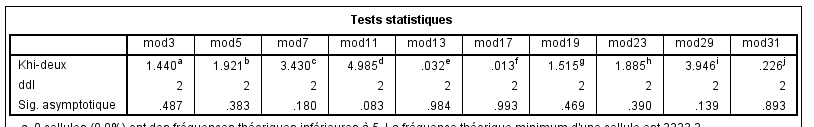
EDITED ANSWER. I'm notnow quite sure that this is true for every odd prime $p$. Using the list available on the OEIS (A055507), it appears that when you perform a $\chi^2$ test, for each prime $p=3,5,7,13,17,19,23,29$ and $31$, the finite sequence $\{S(n): n\le10000\}$ is uniformly distributed on $\{0,1,\dots,p-1\}$. The $p$-values are always largely above $.05$. In particular there is a quite strong statistical evidence that there are no congruence classes that appear significantly more often than others.
However, the same analysis for the prime $11$ surprisingly returns as a non$p$-uniform distributionvalue $(p=0.005)$$p=0.005$, but as, H. However his could also be due to the law of small numbersCohen remarked (see comment below), this apparent non-uniformity disappears, when one considers $n\le 10^7$.