This norm is not Gateaux differentiable for $n\ge2$.
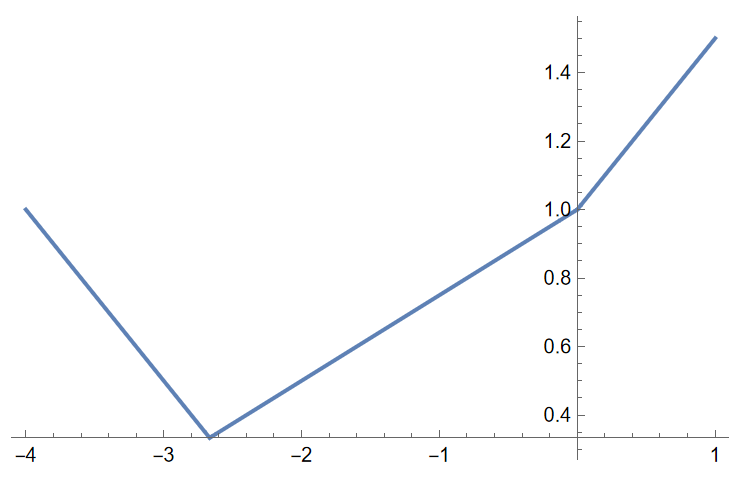
Indeed, let $x:=(2,4,0,0,\ldots)$$x:=(2,4,0,\ldots,0)$ and $u:=(1,1,0,0,\ldots)$$u:=(1,1,0,\ldots,0)$. Then $$g(t):=\|x+tu\|_0=\max(|1+\tfrac t4|,|1+\tfrac t2|) \\ =-(1+\tfrac t2)\,1(t\le-\tfrac83)+(1+\tfrac t4)\,1(\tfrac83<t\le0) +(1+\tfrac t2)1(t>0)$$ for real $t$. So, $g$ is not differentiable at $0$ (and also at $-8/3$). $\quad\Box$
Here is the graph $\{(t,g(t))\colon-4<t<1\}$: