Here is J. Alper's definition of good moduli spaces. 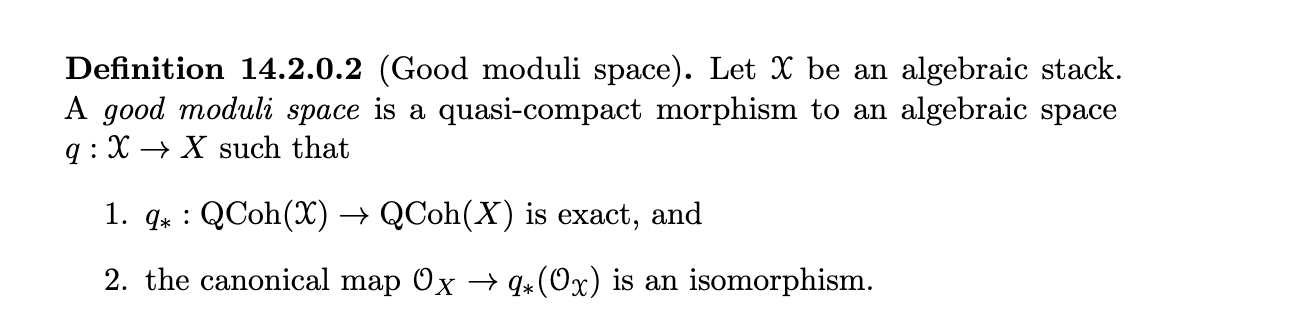
Consider in characteristic zero. Then we see that the classifying stack of any non-reductive group $H$ does not have a good moduli space. In particular the natural morphism $p:[*/H]\to *$ is not a good moduli space. This is because $p_*$ sends a $H$-representation $V$ to $V^H$, which is not right exact.
Good moduli spaces follow from good categorical quotients in GIT, where non-reductive groups do not play a role. However, there are actions with obvious nice quotients, for example the trivial action $H\curvearrowright *$ and the left multiplication $H\curvearrowright H$. One of them, $[*/H]$, does not have a good moduli space, and the other, $[H/H]$, does have one.
My questions are as follows.
- Do people define other terminologies so that $[*/H]\to*$ has a name?
- Why reductivity of stabilizers of (closed) points so desirable?
