Define the smooth map $f : \mathbb{R} \rightarrow \mathbb{R}$ by $f(x) := \frac{1-\cos(x)}{x^2} = -\sum\limits_{k=1}^\infty \frac{(-1)^k}{(2k)!} x^{2k-2}$.
I am looking for a nice bound on $|f^{(n)}(x)|$ for any $n \in \mathbb{N}_0$ and $x \in \mathbb{R}$. Some very preliminary simulations seem to suggest something like $|f^{(n)}(x)| \leq \min(\frac{1}{2},\frac{2}{x^2})$.
This seems easy at first glance, since we can just give an explicit formula for the $n$-th derivative with \begin{align*} & f^{(n)}(x) = \sum\limits_{k=0}^n {n \choose k} \partial_x^k[1-\cos(x)] \, \partial_x^{n-k}[x^{-2}] \ , \end{align*} which is a relatively simple formula, but using this formula to explain the behaviour for $x\rightarrow 0$ is already difficult.
Here is a plot containing $f$(blue), $f^{(1)}$(orange), $f^{(2)}$(green), $f^{(3)}$(purple), $f^{(4)}$(brown) and $\min(\frac{1}{2},\frac{2}{x^2})$(red):
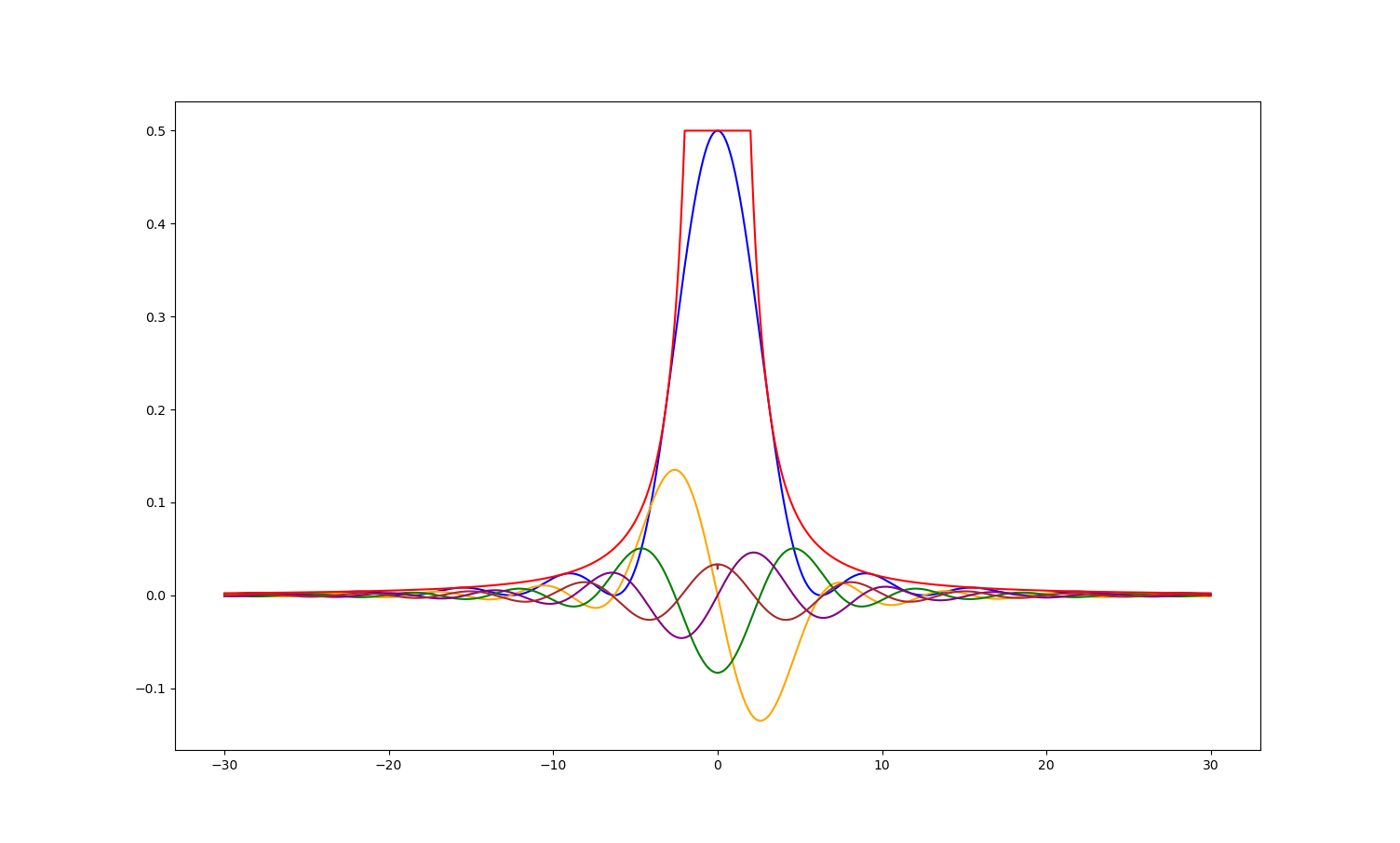
Any help is much apprechiated!
Edit: Thank you for all the helpful answers! I used the idea by Fedor Petrov to adjust your bounds to my needs. I arrive at \begin{align*} & |f^{(n)}(x)| \leq \min\Big( \frac{1}{(n+1)(n+2)}, \frac{6}{x^2} \Big) \ . \end{align*} Since the first bound $|f^{(n)}(x)| \leq \frac{1}{(n+1)(n+2)}$ was already shown by multiple answers and since $f$ is symmetric, it suffices to show $|f^{(n)}(x)| \leq \frac{5}{x^2}$$|f^{(n)}(x)| \leq \frac{6}{x^2}$ for $x \geq \sqrt{6(n+1)(n+2)}$. For $n \geq 2$ we have the following calculations:
Since \begin{align*} & f^{(n)}(x) = \Re\bigg( \int_0^1 (1-t) (it)^n e^{itx} \, dt \bigg) \ , \end{align*} integrating by parts twice yields \begin{align*} & f^{(n)}(x) = \Re\bigg( \Big[ (1-t) (it)^n \frac{-i e^{itx}}{x} \Big]_0^1 - \int_0^1 \frac{(it)^n (n(1-t)-t)}{t} \frac{-i e^{itx}}{x} \, dt \bigg)\\ & = \Re\bigg( 0 + i \int_0^1 \frac{(it)^n (n(1-t)-t)}{t} \frac{e^{itx}}{x} \, dt \bigg)\\ & = \Re\bigg( i \Big[ \frac{(it)^n (n(1-t)-t)}{t} \frac{-i e^{itx}}{x^2} \Big]_0^1 - i \int_0^1 \frac{n(it)^n (n(1-t)-t-1)}{t^2} \frac{-i e^{itx}}{x^2} \, dt \bigg)\\ & = \Re\bigg( i^2 i^n \frac{e^{ix}}{x^2} + \frac{i^2 n^2}{x^2} \int_0^1 \frac{1-t}{t^2} (it)^n e^{itx} \, dt - \frac{i^2 n}{x^2} \int_0^1 \frac{t+1}{t^2} (it)^n e^{itx} \, dt \bigg) \end{align*} and we for $n \geq 2$ get the bound \begin{align*} & |f^{(n)}(x)| \leq \frac{1}{x^2} + \frac{n^2}{x^2} \underbrace{\int_0^1 \frac{1-t}{t^2} t^n \, dt}_{=\frac{1}{n(n-1)}} + \frac{n}{x^2} \underbrace{\int_0^1 \frac{t+1}{t^2} t^n \, dt}_{= \frac{1}{n} + \frac{1}{n-1}} \leq \frac{6}{x^2} \ . \end{align*} It remains to check the cases $n=0$ and $n=1$. We calculate \begin{align*} & |f^{(0)}(x)| = \bigg|\Re\bigg( \int_0^1 (1-t) e^{itx} \, dt \bigg)\bigg| = \bigg|\Re\bigg( \frac{ix-e^{ix}+1}{x^2} \bigg)\bigg| \leq \frac{2}{x^2} \end{align*} and \begin{align*} & |f^{(n)}(x)| = \bigg|\Re\bigg( i \int_0^1 (1-t) t e^{itx} \, dt \bigg)\bigg| = \bigg|\Re\bigg( -i \frac{x+e^{ix}(x+2i)-2i}{x^3} \bigg)\bigg| \leq \frac{1}{x^2} + \frac{4}{x^3} \overset{x \geq 1}{\leq} \frac{5}{x^2} \ . \end{align*}
