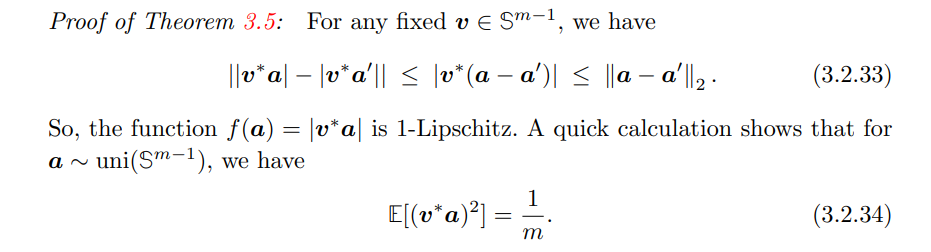
For any fixed $\mathbf v \in \mathbb S^{m-1}$, we have $$||\mathbf v * \mathbf a| - | \mathbf v * \mathbf a' || \le | \mathbf v * (\mathbf a - \mathbf a') | \le \| \mathbf a - \mathbf a' \|_2. \tag{3.2.23}$$$$||\mathbf v^* \mathbf a| - | \mathbf v^* \mathbf a' || \le | \mathbf v^* (\mathbf a - \mathbf a') | \le \| \mathbf a - \mathbf a' \|_2. \tag{3.2.23}$$ So, the function $f(\mathbf a) = | \mathbf v * \mathbf a |$$f(\mathbf a) = | \mathbf v^* \mathbf a |$ is $1$-Lipschitz. A quick calculation shows that for $\mathbf a \sim \operatorname{uni} (\mathbb S^{m-1})$, we have $$\mathbb E [ (\mathbf v * \mathbf a)^2] = \frac 1m. \tag{3.2.24}$$$$\mathbb E [ (\mathbf v^* \mathbf a)^2] = \frac 1m. \tag{3.2.24}$$
I am confused about the Equation 3.2.34, can somebody help me, thanks!
