Let $G$ be a $k$-connected planar triangulation ($k\geq 4$) and let $C$ be a Hamilton cycle of $G$. Then:
Which conditions would be sufficient to assure that every triangle of $G$ has at least one edge belonging to the set of edges induced by $C$?
Or, does there exist at least one Hamilton cycle for every 4-connected planar triangulation or every 5-connected planar triangulation with property in (1)?
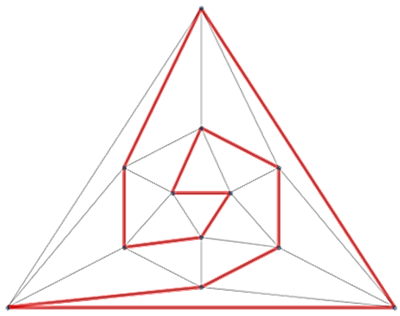
For example: In picture below, not every triangle of $G$ has at least one edge belonging to the set of edges induced by the hamilton cycle $C_1$ (in red+blue)
In Gunnar Brinkmann, Craig Larson, Jasper Souffriau, Nico Van Cleemput - Hamiltonian cycles and triangulations
However, there is ain the next Figure, all triangles contains an edge belonging to the Hamilton cycle without such a property.$C_2$ (in red):