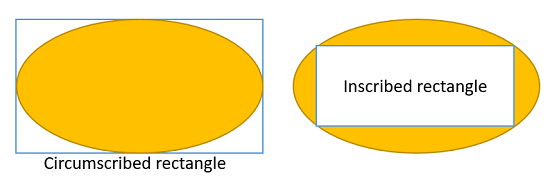
We say a rectangle has orientation $\theta$ if the vector from its center to the middle of its shortest side (parallel to the longest side) has some angle $\theta$ with X axis.
Consider a planar convex region $C$ fixed in $\mathbb{R}^2$. Let us draw $R_{1}$, the smallest rectangle containing $C$ and call the orientation of $R_{1}$, $\theta_1$. Let us also draw the rectangle $R_{2}$, the largest rectangle contained within $C$ and let its orientation be $\theta_2$.
Question: Which planar convex region $R$ maximizes $|\theta_1-\theta_2|$ ?
Note 1: If either rectangle is a square, the $\theta$ values won't be unique and we take the smallest value of $|\theta_1-\theta_2|$ as the orientation difference.
Note 2: The same question can be asked with area replaced with perimeter.
Related: bounds on largest internal rectangle area and considering a simple case: right triangles