A new paper was just posted to the arXiv that develops a polynomial algorithm for some cases of "2-concentric parallel regular polygons." Here are Figs. 1(a) and 5:

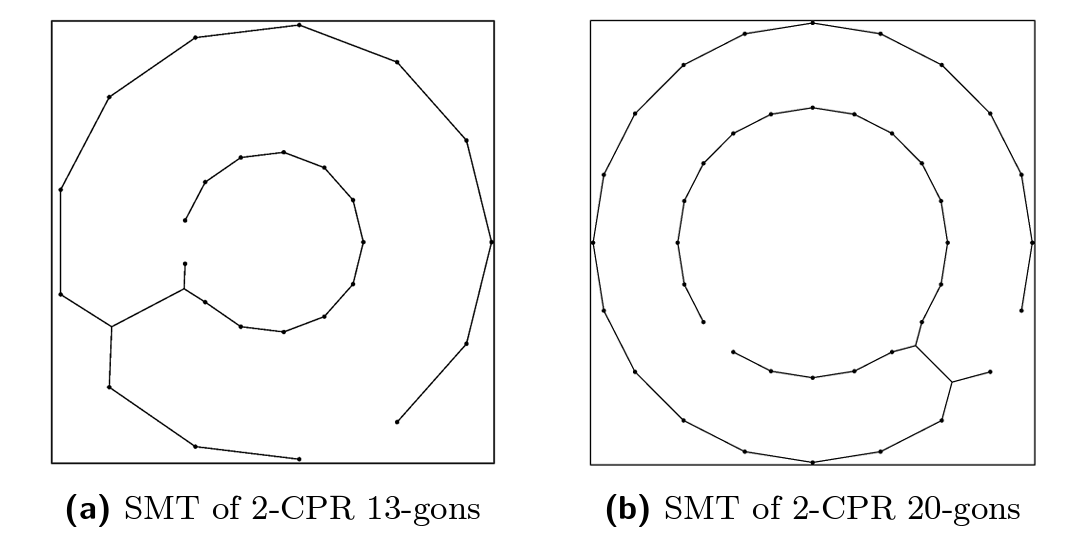
Dhar, Anubhav, Soumita Hait, and Sudeshna Kolay. "Efficient Algorithms for Euclidean Steiner Minimal Tree on Near-Convex Terminal Sets." arXiv:2307.00254 (2023).
They also obtain results for "$f(n)$-almost convex sets."
