The $h$-vector of the (simplicial complex given by the boundary of the polytope dual to the) permutohedron is the sequence of Eulerian numbers $A(n,k)=\{w\in S_n\colon \mathrm{des}(w)=k\}$$A(n,k)=\#\{w\in S_n\colon \mathrm{des}(w)=k\}$.
Example: The (dual of the) 2-dimensional permutohedron is a hexagon, with 6 1-cells, 6 0-cells, and 1 -1-cell. So we compute the $h$-vector by $$\begin{array}{c c} (1,6,6) &= 1 \times (1,2,1)\\ & +4 \times (0,1,1) \\ &+1 \times (0,0,1) \end{array}$$ so that its $h$-vector is $(1,4,1)$.
Meanwhile the $h^*$-vector of the unit hypercube is also given by the Eulerian numbers. This means that the $h$-vector of any unimodular triangulation of the hypercube is the Eulerian numbers, padded with two trailing 0's.
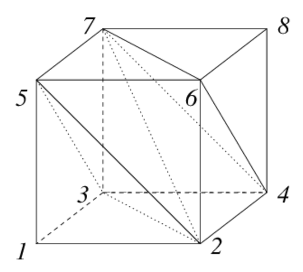
Example: Looking at
we see that a unimodular triangulation of the cube has 6 tetrahedra, 18 triangles, 19 edges, and 8 vertices. So we compute the $h$-vector of this triangulation by $$\begin{array}{c c} (1,8,19,18,6) &= 1 \times (1,4,6,4,1)\\ & +4 \times (0,1,3,3,1) \\ &+1 \times (0,0,1,2,1) \\ &+0 \times (0,0,0,1,1) \\ &+0 \times (0,0,0,0,1) \end{array}$$ so that the $h$-vector is $(1,4,1,0,0)$.
Note: There is a pretty canonical unimodular triangulation of the hypercube whose maximal simplices are $0 \leq x_{w_1} \leq x_{w_2} \leq \cdots \leq x_{w_n} \leq 1$ for all permutations $w = w_1\cdots w_n$.
Question: Is there some deeper geometric connection between a triangulation of the hypercube and the permuotohedron suggested by this numerology?
In terms of what an answer could be: I believe if $\Delta$ is a $(d-1)$-dimensional simplicial complex with $h$-vector $(c_0,\ldots,c_d)$, then $\Delta \ast \mathrm{pt}$ (the cone over $\Delta$, i.e., the result of joining $\Delta$ with a single vertex) has $h$-vector $(c_0,\ldots,c_d,0)$. So theThe simplest thing would be if the triangulation of the cube were the result of coning the permutohedron twice. But I don't think that is quite the case. Still, maybe you can start with this double cone of permutohedron and then do... somethingSo... to get
Question, hopeful version: Does double cone over the permutohedron give a unimodular triangulation. of the hypercube?