Given a vector bundle $E\rightarrow M$, we have a collection of vector spaces $\{E_m\}_{m\in M}$ indexed by elements of $M$. Every vector space gives a Lie algebra $\rm{End}(V)$. This collection of vector spaces $\{E_m\}_{m\in M}$ would also give a collection of Lie algebras $\{\rm{End}(E_m)\}_{m\in }$ indexed by elements of $M$. Even though fibers of vector bundlebundles are vector spaces, it is usually not the case that the fiber of Lie algebroid is a Lie algebra. So, it is unlikely that this collection $\{\rm{End}(E_m)\}_{m\in M}$ of Lie algebras indexed over elements of $M$ would give an(y) interesting Lie algebroid over $M$. I would like to know if this is of any interest, any comments are welcome.
If we focus on subsetsubsets of ${\rm End}(\Gamma(M,E))$ that are $C^\infty(M)$-linear then there is the endomorphism bundle. Otherwise, it is not clear what could be a choice. Note that we should also be able to assign a map from that subspace of sections to the sections of the tangent bundle. It is not clear how do you assign a vector field on $M$ for a $C^\infty(M)$-linear map of sections.
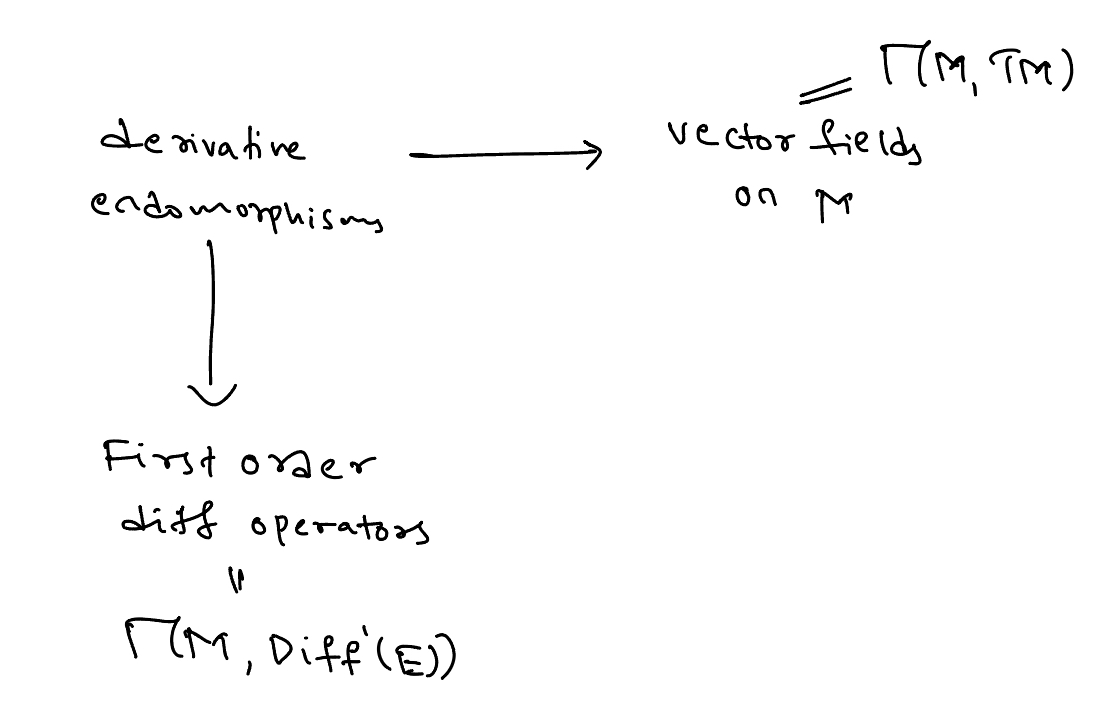
If we denote this subset of derivative endomorphisms by $S$, we have a map $S\rightarrow \Gamma(M,TM)$, sending $D$ to $D_X$ (at this point, I do not know if for a given $D$, there can be more than one vector fields $D_X$ associated to $D$). Now, we need to know if this $S$ is the space of sections of some vector bundle.
This construction of vector bundlebundles is done in two steps. Firstly, we look at a more general notion of first order differential operators (see Regarding first order differential operator and derivative endomorphism). It is "understood" that, this first order differential operators are sections of vector bundle ${\rm Diff}^1(E)\rightarrow M$ (if you know any place that gives some details about this bundle, please leave references in comments).
This set of derivative endomorphisms is a subset of first order differential operators that has something to do with vector fields, as in the following diagram,
When everWhenever we want something common between two properties, we take "intersection". An appropriate notion of "intersection" is the pullback. The question is pullback with respect to which manifolds and which maps. The candidate for that is ${\rm{Hom}}(T^*M, {\rm End}(E))$. They define some bundle morphisms ${\rm Diff}^1(E)\rightarrow {\rm{Hom}}(T^*M, {\rm End}(E))$ and $TM\rightarrow {\rm{Hom}}(T^*M, {\rm End}(E))$ and declare the pullback to be the Lie algebroid of derivations $\mathcal{D}(E)\rightarrow M$ as in the following diagram,

