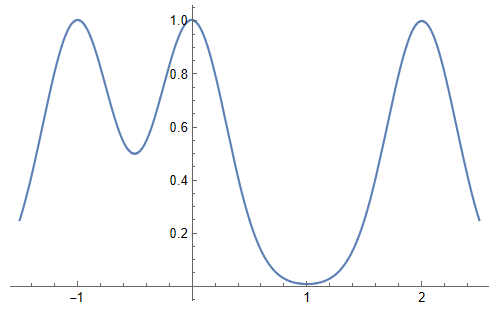
In general, it is not true at all that $x^*_h\to x_1$ as $h\downarrow0$. Indeed, suppose that $n=3$, $x_1=2$, $x_2=0$, $x_3=-1$.
Then for small $h>0$ there will be a critical point $x^*_h$ close to the midpoint $1$ between $x_1=2$ and $x_2=0$, with $x_1=2$ being the closest to $x^*_h$ among the points $x_1,x_2,x_3$ -- because $f'(1)=-2Ce^{-2/h^2}/h^2<0$. However, $x^*_h-x_1$ will be close to $1-2=-1$ and not to $0$.
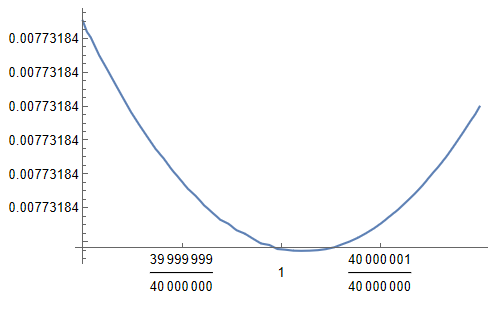
For an illustration, here isare the graphgraphs $\{(x,f(x))\colon x\in[-1.5,2.5]\}$ and $\{(x,f(x))\colon x\in[1-\frac12\,10^{-7},1+\frac12\,10^{-7}]\}$ for $h=3/10$: