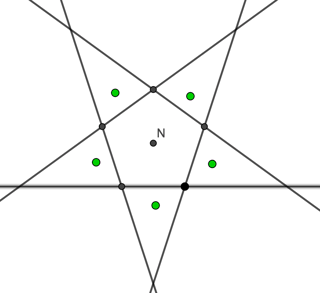
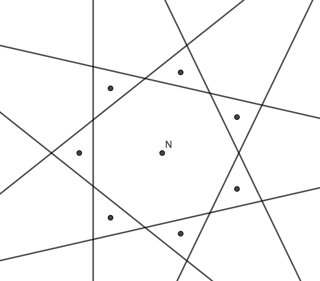
You can pack as many vectors $m_i$ and $\mu_i$ as you want in $\mathbb{S}^2$. Below I give an example with $5$ points: consider $5$ points $m_i$ forming a regular pentagon parallel to the equator and a bit below it. Below is a figure showing approximately how the north pole $N$ and the five geodesics perpendicular to the $m_i$ look like (note that $\langle N,m_i\rangle<0$ for all $i$).

Now choose $\mu_i$ in the positions of the green points, so that $\langle \mu_i,m_i\rangle>0$ for all $i$ but for $j\neq i$ we have $\langle \mu_i,m_j\rangle<0$.

You can pack as many vectors $m_i$ and $\mu_i$ as you want in $\mathbb{S}^2=\{x^2+y^2+z^2=1\}\subseteq\mathbb{R}^3$. I leave below an example with $7$ points that generalizes to any finite number of points.

Consider points $m_1,\dots,m_7$ forming a regular heptagon in $\mathbb{S}^2\cap\{z=-0.01\}$. Below is a figure showing how a small neighborhood of the north pole $N=(0,0,1)$ in $\mathbb{S}^2$ would look like, with the lines being the sets $\{v\in\mathbb{S}^2;\langle m_i,v\rangle=0\}$, for $i=1,\dots,7$. Note that $\langle m_i,N\rangle<0\;\forall i$.

Now choose $\mu_i$ in the positions of the seven points from the figure, so that $\langle \mu_i,m_i\rangle>0$ for all $i$ but for $j\neq i$ we have $\langle \mu_i,m_j\rangle<0$.