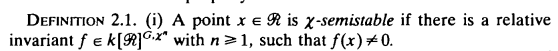Suppose $G$ is a linearly reductive algebraic group acting linearly on a finite dimensional vector space $V$ over $\mathbb{K}$ (algebraically closed)$\mathbb{C}$. This induces an action on the coordinate ring $\mathbb{K}[V]$$\mathbb{C}[V]$ (see here). Let $\chi:G\rightarrow\mathbb{K}^*$$\chi:G\rightarrow\mathbb{C}^*$ be a multiplicative character of $G$. An element $f\in\mathbb{K}[V]$$f\in\mathbb{C}[V]$ is said to be relative invariant of weight $\chi$ if $$(g\cdot f)(x):=f(g^{-1}\cdot x)=\chi(g)f(x),\text{ for every }x\in V\text{ and }g\in G.$$ (You can also see here)
Suppose $a\in V$ is a $\chi$-semi-stable point. Hence, by definition, there exists a relative invariant, say $p\in\mathbb{K}[V]$$p\in\mathbb{C}[V]$, of weight $\chi^n$, for some $n\in\mathbb{N}$, (see the full definition below)
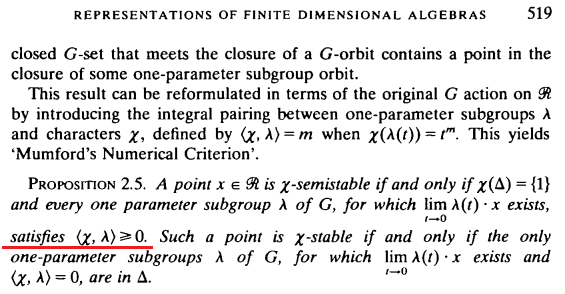
Suppose there exists a one-parameter subgroup $\lambda$ of $G$ such the limit $\lim_{t\rightarrow 0}\lambda(t)\cdot a$ exists. Let $\lim_{t\rightarrow 0}\lambda(t)\cdot a=b$. By the proposition below
We know that $\chi(\lambda(t))=t^{m}$, for some non-negative integer $m$. Since $\chi$ is a multiplicative character, $\chi(\lambda(t)^{-1})=t^{-m}$.
Since $p$ is a polynomial, it's a continuous function. Therefore, $$\lim_{t\rightarrow 0}t^{-nm}p(a)=\lim_{t\rightarrow 0}\chi^n(\lambda(t)^{-1})p(a)=\lim_{t\rightarrow 0}p(\lambda(t)\cdot a)=p(\lim_{t\rightarrow 0}\lambda(t)\cdot a)=p(b)$$ But if $m>0$ then the left hand side diverges. Therefore, $m=0$.
What I'm confused about is that this would mean $\langle\chi,\lambda\rangle$ is always $0$, but I don't think this is true. I don't understand where the mistake is.
The reference for above definition and proposition is- A. D. King Moduli of representations of finite dimensional algebras