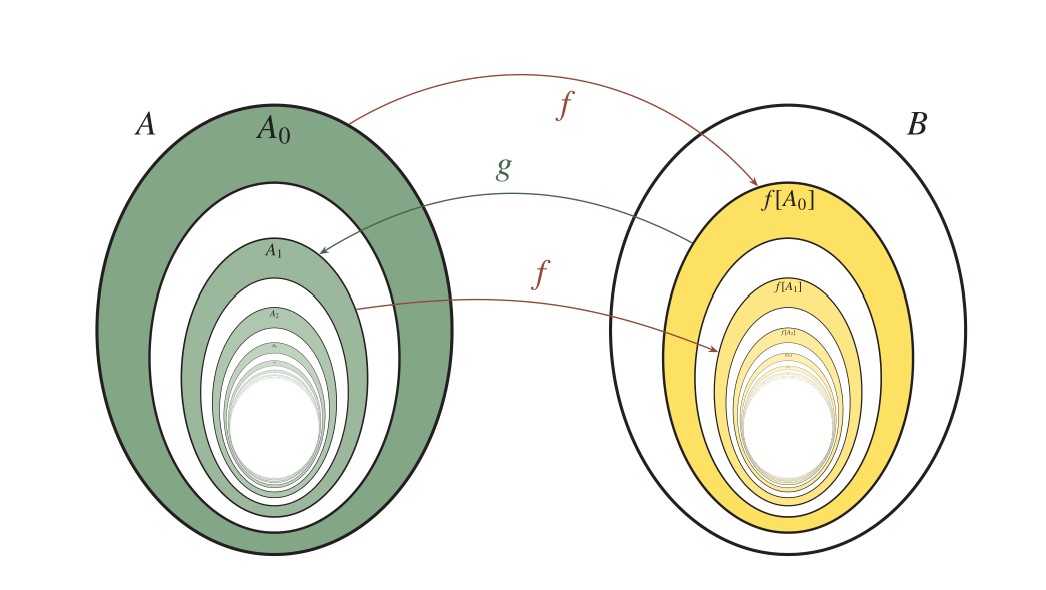
Another classic example is the Schröder-Cantor-Bernstein theorem.
Theorem. If a set $A$ injects into $B$ and $B$ injects into $A$ then there is a bijection.
If AC holds, then this is nearly trivial, since if $A$ and $B$ are well orderable, then the minimal ordinals would have to be the same, giving a bijection.
But there is a more constructive proof (one not using AC), stitching together pieces of the injections as illustrated in the following figure.
Historically, the distinction between the proofs is important, and actually the history of the theorem and who proved what when is quite complicated. See the Wikipedia entry.