A magic square is one in which the sum of the numbers in each row, column, and both main diagonals is the same. The numbers in the magic square can only be 1 to 9.
a 3x3 magic square example:
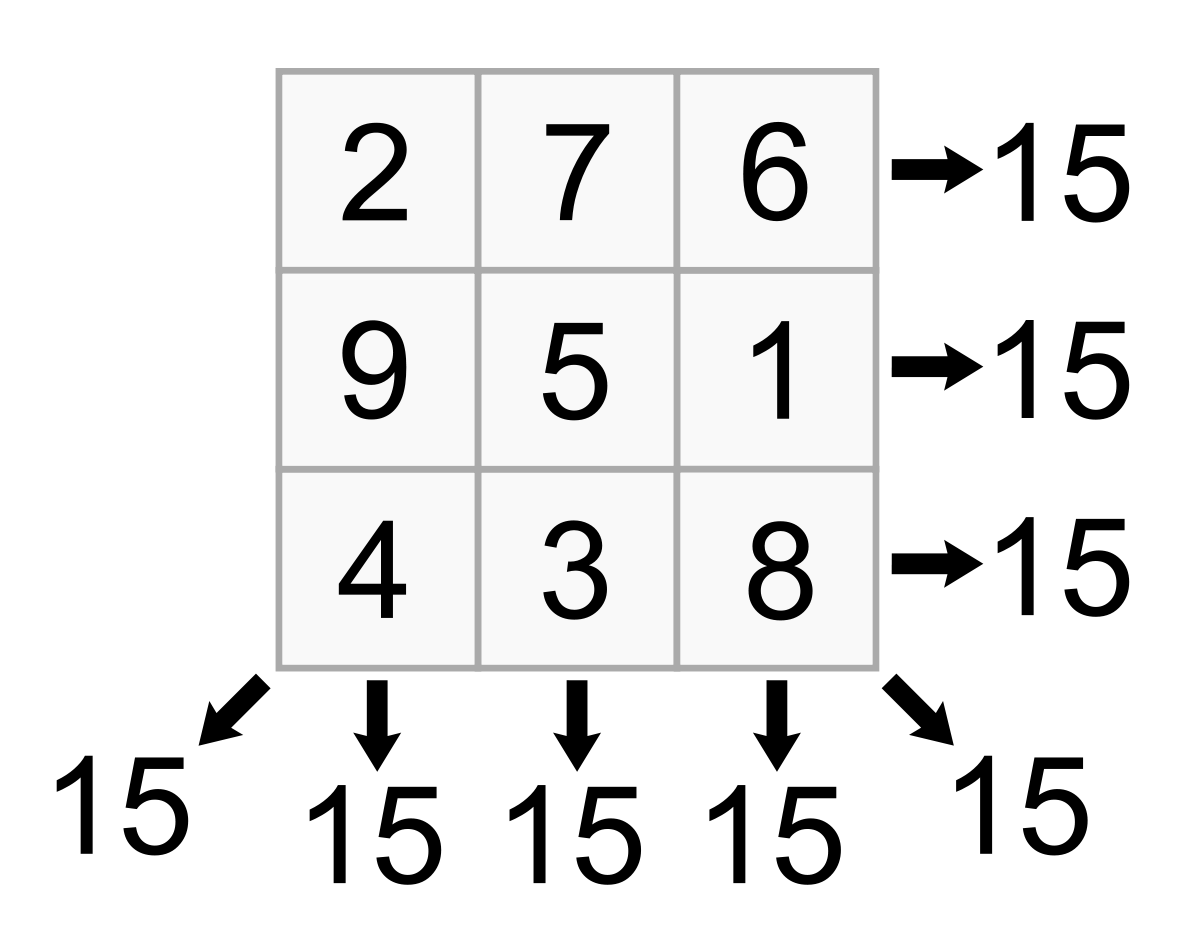
There are 8 combinations of a 3x3 magic square.
A white Rubik's cube has 6 sides. There are 9 spaces on each side for numbers from 1 to 9, without color.

If we fill each side of a white Rubik's cube with a magic square, how many combinations can be filled on this Rubik's Cube?
