Return to Answer

No.

E.g., take $a=4$ and $b=27/8$. Then the positive roots of $g$ are $x_1\approx0.338$, $x_2\approx0.826$, $x_3\approx3.78$, and $f(x_1)\approx13.6$ and $f(x_3)\approx9.72$, so that $x_1$ is not a global minimizer of $f$ on $(0,\infty)$.

For an illustration, here are graphs of $f$ (blue) and $g$ (red):

No.

E.g., take $a=4$ and $b=27/8$. Then the positive roots of $g$ are $x_1\approx0.338$, $x_2\approx0.826$, $x_3\approx3.78$, and $f(x_1)\approx13.6$ and $f(x_3)\approx9.72$, so that $x_1$ is not a global minimizer of $f$ on $(0,\infty)$.

For an illustration, here are graphs of $f$ (blue) and $g$ (red):

No.

E.g., take $a=4$ and $b=27/8$. Then the positive roots of $g$ are $x_1\approx0.338$, $x_2\approx0.826$, $x_3\approx3.78$, and $f(x_1)\approx13.6$ and $f(x_3)\approx9.72$, so that $x_1$ is not a global minimizer of $f$ on $(0,\infty)$.

No.

E.g., take $a=4$ and $b=27/8$. Then the positive roots of $g$ are $x_1\approx0.338$, $x_2\approx0.826$, $x_3\approx3.78$, and $f(x_1)\approx13.6$ and $f(x_3)\approx9.72$, so that $x_1$ is not a global minimizer of $f$ on $(0,\infty)$.

For an illustration, here are graphs of $f$ (blue) and $g$ (red):

Yes.

Indeed, $f(0+)=\infty=f(\infty-)$. So, the smooth function $f$ attains a minimum at some critical point of $f$ in $(0,\infty)$, that is, at a root $x\in(0,\infty)$ of $g$, where $g(x)$ is of the same sign as $f'(x)$ for each $x>0$.

The function $g$ is concave on $(0,a/2]$ and convex on $[a/2,\infty)$. Also, $g(0)=-1<0$, $g'(0)=b>0$, and $g(\infty-)=\infty$. So, either (i) $g$ has a unique root in $(0,\infty)$ or (ii) $g$ has exactly two roots $u,v$ such that $0<u<v<\infty$.

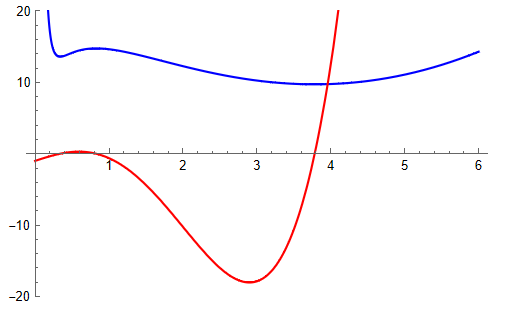
We only have to consider case (ii), in which $g<0$ on $(0,u)$ and $g>0$ on $(u,v)$. So, $f'<0$ on $(0,u)$ and $f'>0$ on $(u,v)$. So, $f$ is decreasing on $(0,u)$ and increasing on $(u,v)$. So, the minimizer of $f$ on $(0,\infty)$ is the smallest root, $u$, of $g$ on $(0,\infty)$. $\quad\Box$

No.

E.g., take $a=4$ and $b=27/8$. Then the positive roots of $g$ are $x_1\approx0.338$, $x_2\approx0.826$, $x_3\approx3.78$, and $f(x_1)\approx13.6$ and $f(x_3)\approx9.72$, so that $x_1$ is not a global minimizer of $f$ on $(0,\infty)$.