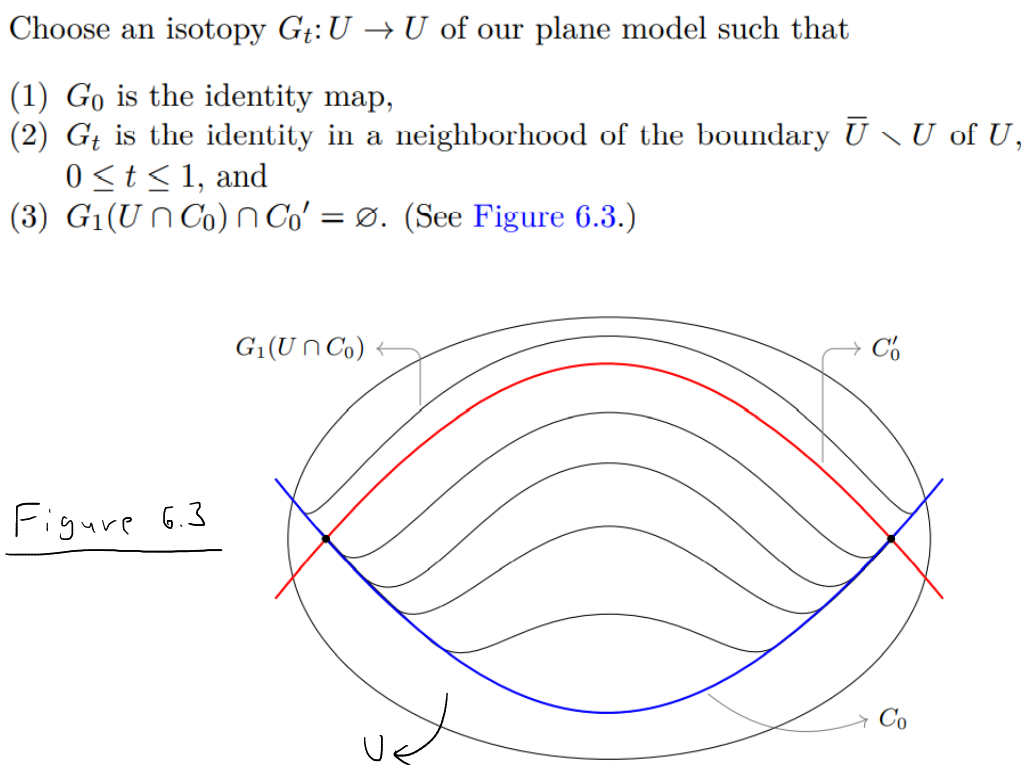
I'm estudyingstudying the H-Cobordism theorem following the Lectures of John Milnor, and in the proof of the Whitney trick for cancel pairs of self-intersection points I have the next problem with an isotopy wichwhich is used without formal definition:
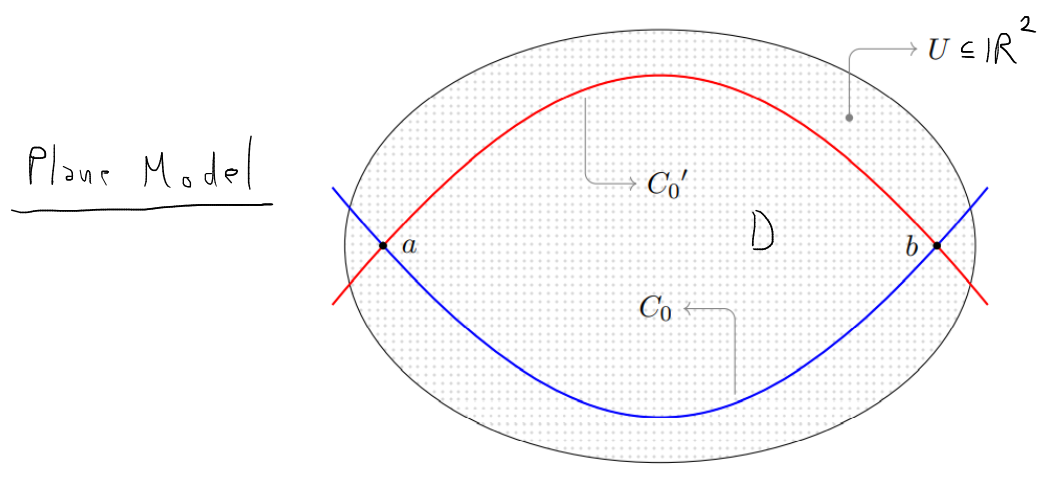
Considering the next plane model composed of two curves $C_0$ and $C'_0$ wich intersect transversally at two points $a$ and $b$ and enclose a disk $D$ in a domain $U$ of $\mathbb{R}^2$.
Milnor says the following:
What is the best way to formalize this isotopy?