(This answer was posted before the convexity condition on the curve $\gamma$ was added to the question)
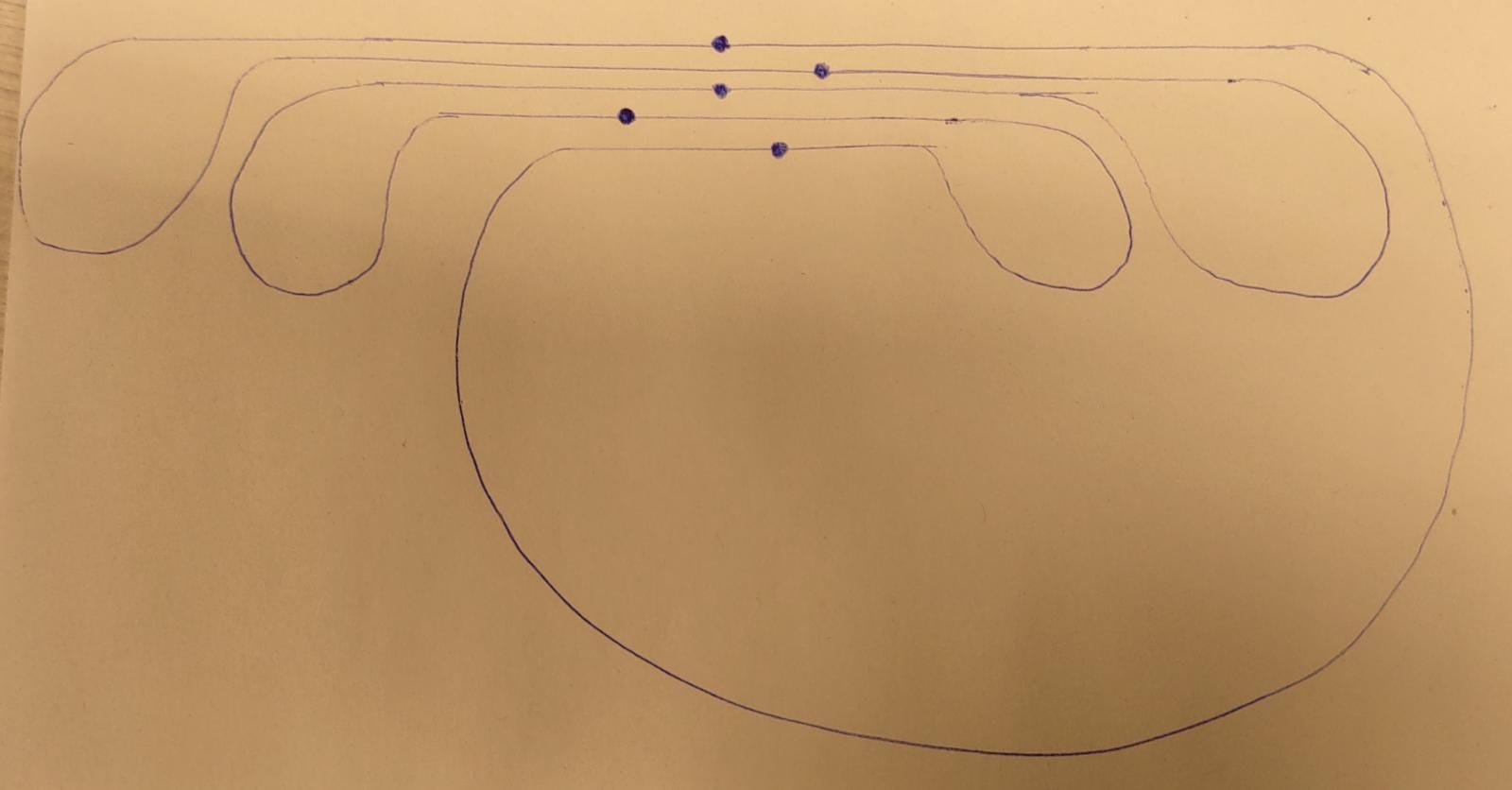
Suppose you have any finite set of points in $\mathbb{R}^2$, and rotate $\mathbb{R}^2$ so that they have pairwise distinct $y$-coordinates. Then we can create curves with arbitrarily low curvature passing through all the points. Here is an idea of what they would look like:
You can make the curves from the sides arbitrarily large, thus making their curvatures arbitrarily small.