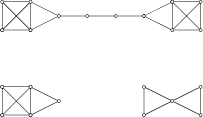
Please let me know if the following graphs popped up in some problems.
Each of these graphs is described by 5 integers $n_1\geqslant k_1$, $n_2\geqslant k_2$, $l\geqslant 0$. We take two complete graphs $K_{n_1}$ and $K_{n_2}$ and a path $P_l$ of length $l$, the left endvertex of $P_l$ is connected to $k_1$ vertices of $K_{n_1}$ and and the right endvertex of $P_l$ is connected to $k_2$ vertices of $K_{n_2}$. In addition we assume that if $n_i>0$ then $k_i>0$, so the obtained graph is connected.
Here three examples for
- $n_1=4,k_1=2,l=3,k_2=2,n_2=4$;
- $n_1=4,k_1=2,l=0,k_2=0,n_2=0$;
- $n_1=2,k_1=2,l=0,k_2=2,n_2=2$.
These graphs encode solutions of certain problem in metric geometrycertain problem in metric geometry related to the so-called graph comparison.