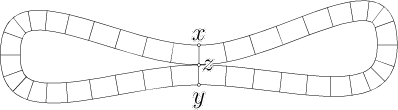The answer is yes.
The map $F$ is defined in $N \times [0,\varepsilon]$; plus it is smooth. Further, your condition on second fundamental form and curvature imply that it is regular in $N \times [0,10\cdot L]$, where $L=L(C_1,C_2,\varepsilon)>0$.
Suppose $F$ is not injective in $N \times [0,L]$. Let $\ell$ be the smallest value such that $F$ is not injective in $N \times [0,\ell]$. In this case there are two points $x,y\in N$ such that $z=\exp_x(\ell \cdot n_x)=\exp_y(\ell\cdot n_y)$. Since $\ell$ is minimal, we have that geodesics $[zx]$ and $[zy]$ point in the opposite directions. It follows that $y=\exp_x(2\cdot \ell\cdot n_x)$ and therefore $\exp_x(10\cdot L n_x)$ is undefined --- a contradiction.