This problem is motivated by the edge states that sometimes appear (and sometimes not) at the level of Huckel hamiltonians for $\pi$-conjugated benzenoid hydrocarbons. If this sentence is cryptic, forget it for the moment, but for those familiar with the basic concepts of tight-binding hamiltonians and the like this will be a strong motivation to grasp the interest of the problem.

Consider a bipartite graph $G=(V,E)$ and consider its traceless laplacian, H (a matrix for which the diagonal is of zeros and $H(i,j) = -1$ only if i and j are neighboring vertices, otherwise is zero).

Call $2N$ to the number of vertices of $G$, assumme the spectrum of $G$ is non-degenerate, that $G$ has a even number of vertices ($2N$), that each sublattice (of the bipartite graph) contains the same number of vertices, $N$, and if necessary that the graph is hexagonal (in the sense that every vertex is on a 6-cycle and the are no cycles of smaller order).

I will consider the eigenvector associated to the smallest eigenvalue (in absolute value). If we list the eigenvalues from smallest to largest, this is exactly the $N$-th eigenvalue. Call this eigenvector $f: G \rightarrow \mathbb{R}$ and assume it normalized, $\sum_{g \in G} \vert f(g)\vert ^2 = 1.$

Now I am interested in some quantity that characterizes how delocalized $f$ is over $G$. It could be Shannon entropy, $S(f) = \sum_{g \in G} f(g) \log f(g)$ or something like $U(f) = \sum_{g \in G} \vert f(g) \vert ^4$ (probably intuitivily speaking more relevant for the connection to physics because this $U(g)$ is basically the electrostatic repulsion in a $\pi$-orbital).

Let me show you a couple of examples (fulfilling the conditions I mentioned above) that I calculated numerically: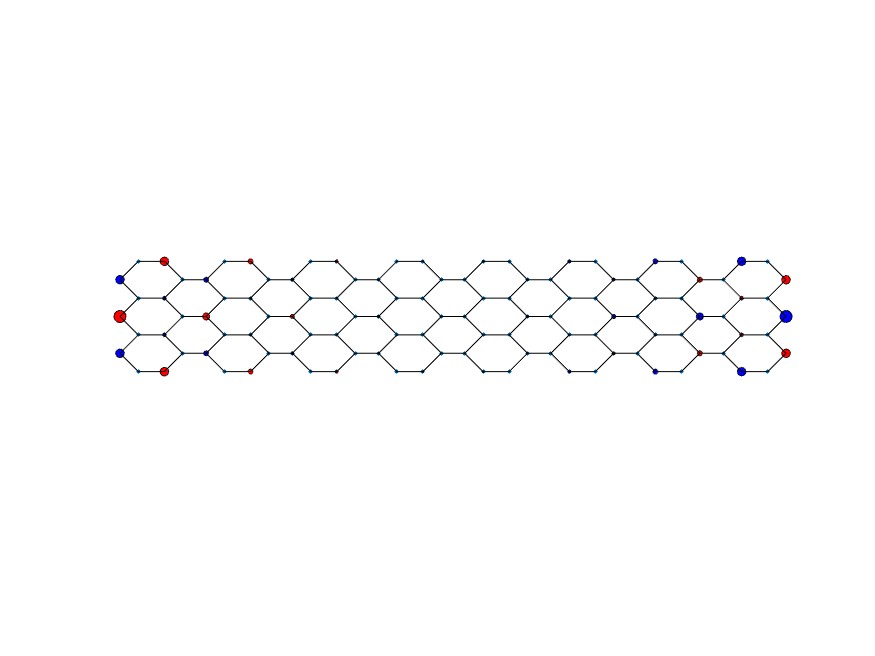

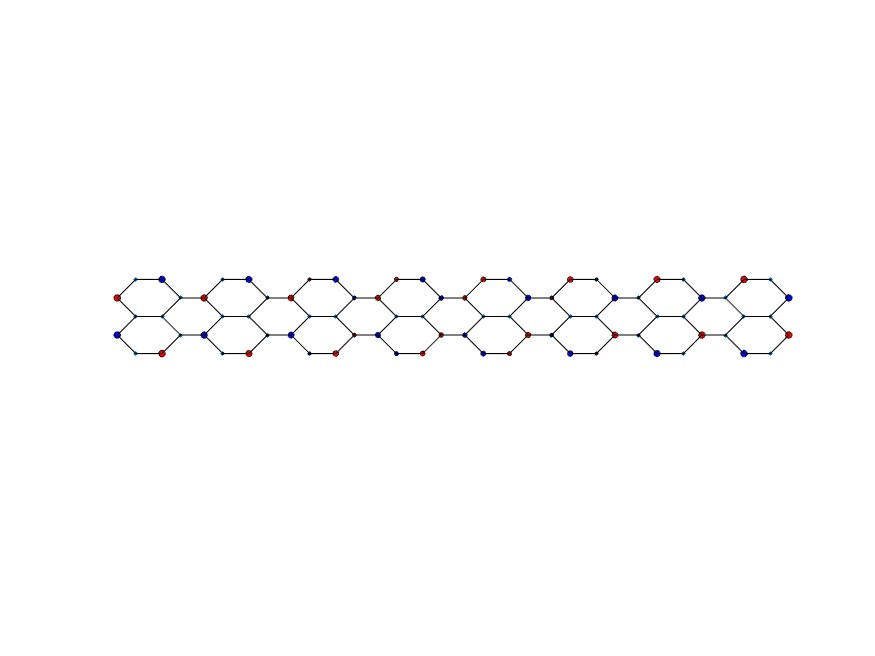
As you can see, the first one produces an f that is much more localized. If you increase the lenghts of these graphs horizontally, you will observe the same phenomenon (I have checked this for huge graphs). Now the question is, why does it happen? The only difference is that in the graph above there is a third row of hexagons.

I thought I could relate this to the rank of the matrices connecting vertices of degree 2 and 3 of the two sublattices, but I calculated the correlation coefficients of the entropies and these ranks and there's nothing there, so it has to be something more subtle.

I know that the question is a bit vague, but, all in all I would say I am looking for something like a conceptual understanding, sort of a criterion in terms of the vertices of $G$ that helps illuminate how can it be that such a simple difference changes the entropy of the eigenvector so dramatically.