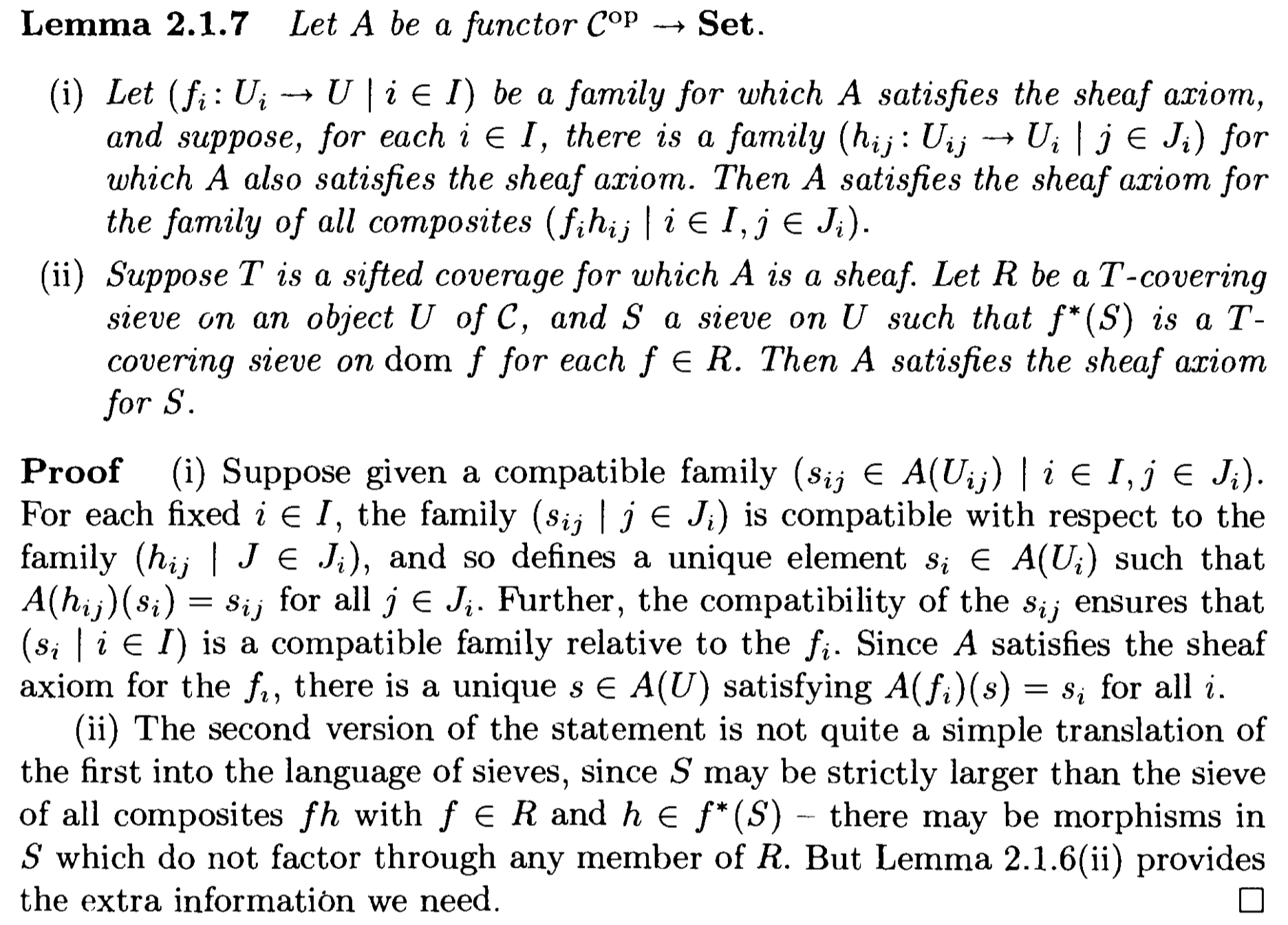I don't understand the proof of (ii) in the Johnstone's Elephant:
Lemma 2.1.6 is:
Now consider $\bigcup_{f \in R} f \circ f^*(S)$. This is my notation for the sieve Johnstone references in the proof of Lemma 2.1.7(ii). The argument of the proof seems to be the following: By Lemma 2.1.7(i), we know that $A$ is a sheaf on $\bigcup_{f \in R} f \circ f^*(S)$. Now since $\bigcup_{f \in R} f \circ f^*(S) \subseteq S$ we apply Lemma 2.1.6(ii) and you are done. But this doesn't make sense, as there is no reason that $\bigcup_{f \in R} f \circ f^*(S)$ is a covering sieve, as we are dealing with an arbitrary sifted coverage here, and Lemma 2.1.6 requires that that it is a covering sieve.
What I was thinking was that we needed then to show that $R \subseteq S$. But that doesn't seem true. Maybe we need to leverage the knowledge that $f^*(S)$ is a covering sieve? I don't see how though.
I am also aware that Lemma 2.1.7(i) is wrong as stated, thanks to this mathoverflow answeranswer but this doesn't change the question.