In Go (Weiqi), two players take turns placing stones on the vacant points of a board. Once placed, stones can only be removed from the board if a stone or a group of stones are surrounded by their opponents on all orthogonally adjacent points, in which case the stone or group is captured.
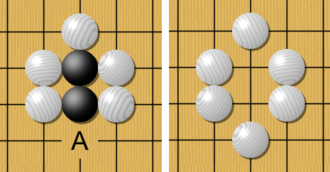
Now we consider a variant of the game. A black stone is put on the center of an empty 19*19 Go board, and then the white player puts its stones on the vacant points. Different from traditional rules, the black will not put any other stones on the chessboard. Instead, he can move his unique stone to all orthogonally adjacent points on the board at will, as long as there is no white piece on the target point.
When the black moves one step, his opponent will take turns placing a white stone on the vacant points to intercept the black, until it is surrounded by white stones on all orthogonally adjacent points, in which case the black stone is captured and the white wins. If the black stone makes it to flee to the edge of the chessboard without being captured, the black will win.
Then my questions are:
- How many white stones at least are needed to capture the only black stone? Or the black player is destined to win the game?
- What if the board is infinite without the edge? Would the black be destined to win in that case?
