$X$ is a nonempty convex subset of $\mathbb{R}^n$ whose element is $x=\left(x_1,...,x_n\right)$.
The theorem is as follows.
If for each $x\in X$, there is an $i \in \left\{1,...,n\right\}$ such that $x_i>0$, then there exists $\left(\lambda_1,...,\lambda_n\right)$ where $\lambda_i \geqslant 0$ for all $i$ and $\sum_{i=1}^n \lambda_i=1$, such that $\lambda \cdot x \geqslant 0$ for all $x\in X$ and $\lambda \cdot x>0$, for some $x \in X$.
I was wondering how to prove it. "$\geqslant 0$ for all $x$" should be easy. But I got stuck in "$>0$ for some $x$". I failed to use the proper separation theorem (Theorem 11.3 in Rockafellar (1970)).
Thank you very much!
This was cross-posted at https://math.stackexchange.com/questions/4504475/how-to-prove-this-corollary-of-hyperplane-separation-theorem?noredirect=1#comment9461236_4504475
Some thoughts:
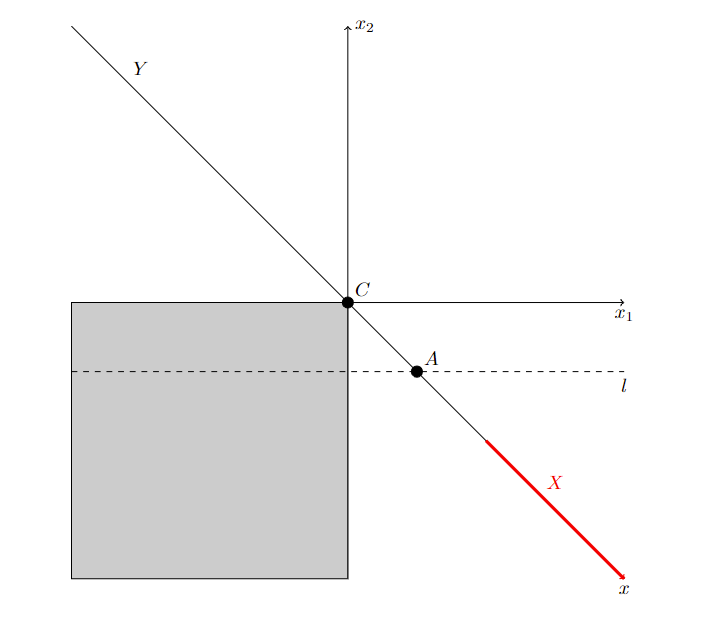
The separation theorem only states the existence of separating hyperplane, but what we need here is a separating hyperplane with some additional properites. An illustration is below.
X is the red line segment. The separating hyperplane we want is $l_2$. But the weak separating theorem may give us $l_1$. I tried to circumvent this by considering the separation of $X$ and the origin. Then the proper separating theorem rules out $l_1$. But it may give us $l_3$, which does not work. Maybe one soluiton is to prove that whenever we have $l_3$, we can construct $l_2$?