A knot in $S^3$ is called a smoothly slice knot if it bounds a smoothly embedded 2-disk in $D^4$. Every ribbon knot is known to be a smoothly slice knot, and there are known some nontrivial smoothly slice knots: https://en.wikipedia.org/wiki/Slice_knot#Examples.
The Stevedore knot (https://en.wikipedia.org/wiki/Stevedore_knot_(mathematics) is an example of a nontrivial knot which is smoothly slice, and the figure below shows that we can obtain the zero-framed Stevedore knot by blowing down a certain link of unknots.
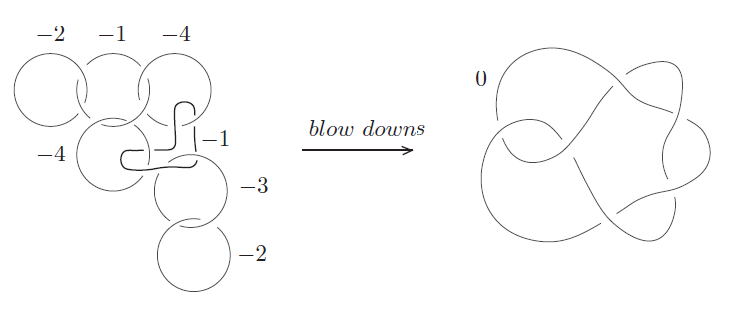
My question is the following: Similarly, is there another example of a 0-framed smoothly slice knot that can be obtained by blowing down successively a link of unknots?
Edit: Can we obtain such a link with the following additional assumptions?
the unknots in the link are negatively-weighted (I think this will hold automatically),
the link has no isolated unknots,
and any two of the unknots of the link are either unlinked or form a Hopf link as in the figure (maybe this is too much to hope).
