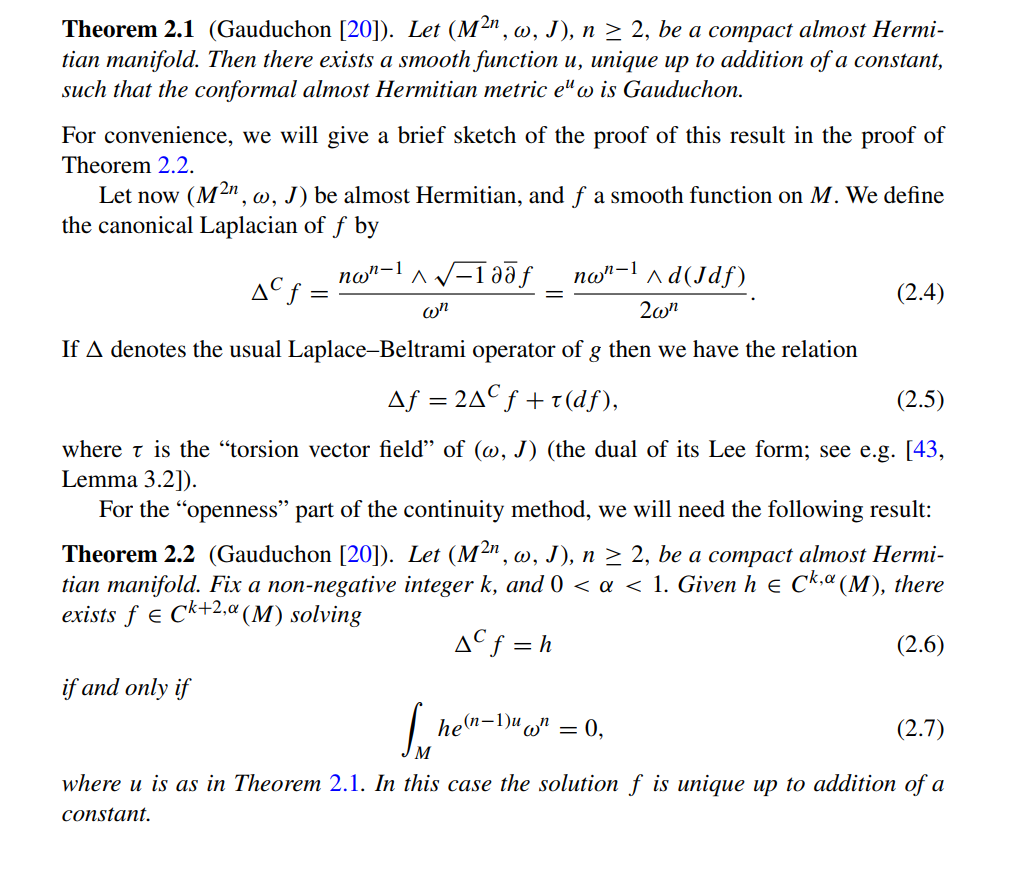
For your first question, note that (let $\omega$ be the Hermitian form)
$$\int_M\Delta_c(f) \omega^n=\int_M(\mathrm{tr}_\omega \sqrt{-1}\partial \overline{\partial}f)\omega^n=n\int_M \sqrt{-1}\partial \overline{\partial}f \wedge \omega^{n-1}$$
Using Stoke's formula, we deduce that
$$\int_M \partial \overline{\partial}f \wedge \omega^{n-1}=\int_M \overline{\partial}f \wedge \mathrm{d}\omega^{n-1}=\int_M \overline{\partial}f \wedge \partial \omega^{n-1}=\int_M f \wedge \partial \overline{\partial} \omega^{n-1}$$
Then $$ \int_M\Delta_c(f) \omega^n=n \int_M f \wedge \sqrt{-1}\partial \overline{\partial} \omega^{n-1} $$
And $\int_M\Delta_c(f) \omega^n=0$ for all $f$ iff $\partial \overline{\partial} \omega^{n-1}=0$. Such metric is called a $\textbf{Gauduchon metric}$.
The answer to your second question is positive and it was first proved by Gauduchon in "Le théorème de l'excentricité nulle"Le théorème de l'excentricité nulle. C. R. Acad. Sci. Paris 285, 387–390 (1977)".
A more accessible reference is "The Monge–Ampère equation for non-integrable almost complex structures""The Monge–Ampère equation for non-integrable almost complex structures" by Chu, Tosatti and Weinkove. They proved the results in Theorem 2.1, Theorem 2.2 in their paper (for almost Hermitian manifolds):